Advertisements
Advertisements
प्रश्न
The line 4x − 3y + 12 = 0 meets x-axis at A. Write the co-ordinates of A. Determine the equation of the line through A and perpendicular to 4x – 3y + 12 = 0.
उत्तर
For the point A (the point on x-axis), the value of y = 0.
4x – 3y + 12 = 0
`=>` 4x = –12
`=>` x = –3
Co-ordinates of point A are (–3, 0)
Here, (x1, y1) = (–3, 0)
The given line is 4x – 3y + 12 = 0
3y = 4x + 12
`y = 4/3x + 4`
Slope of this line = `4/3`
∴ Slope of a line perpendicular to the given line
= `(-1)/(4/3)`
= `(-3)/4`
Required equation of the line passing through A is
y − y1 = m(x − x1)
`y - 0 = (-3)/4 (x + 3)`
4y = −3x − 9
3x + 4y + 9 = 0
APPEARS IN
संबंधित प्रश्न
Find the slope and y-intercept of the line:
ax – by = 0
Find the slope and y-intercept of the line:
3x – 4y = 5
Is the line 3x + 4y + 7 = 0 perpendicular to the line 28x – 21y + 50 = 0?
Verify that points P(–2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle.
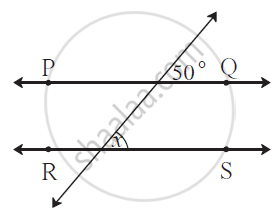
In the figure, line PQ || line RS. Using the information given
in the figure find the value of x.
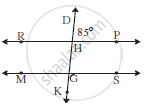
In the adjoining figure line RP ||line MS , line DK is a transversal . If ∠DHP = 85° find ∠RHG and ∠HGS.
Find Equation of CD

Find the equation of the line that has x-intercept = –3 and is perpendicular to 3x + 5y = 1.
A straight line passes through the points P(–1, 4) and Q(5, –2). It intersects x-axis at point A and y-axis at point B. M is the mid-point of the line segment AB. Find:
- the equation of the line.
- the co-ordinates of point A and B.
- the co-ordinates of point M.
If (4,-3) is a point on line 5x +8y = c, find the value of c.
