Advertisements
Advertisements
प्रश्न
The marks obtained by 200 students in an examination are given below :
| Marks | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 | 80-90 | 90-100 |
| No.of students | 5 | 10 | 11 | 20 | 27 | 38 | 40 | 29 | 14 | 6 |
Using a graph paper, draw an Ogive for the above distribution. Use your Ogive to estimate:
(i) the median;
(ii) the lower quartile;
(iii) the number of students who obtained more than 80% marks in the examination and
(iv) the number of students who did not pass, if the pass percentage was 35.
Use the scale as 2 cm = 10 marks on one axis and 2 cm = 20 students on the other axis.
उत्तर १
We construct cumulative frequency table of the given distribution :
| Marks | No. of students (f) | Cumulative Frequency |
| 0-10 | 5 | 5 |
| 10-20 | 10 | 15 |
| 20-30 | 11 | 26 |
| 30-40 | 20 | 46 |
| 40-50 | 27 | 73 |
| 50-60 | 38 | 111 |
| 60-70 | 40 | 151 |
| 70-80 | 29 | 180 |
| 80-90 | 14 | 194 |
| 90-100 | 6 | 200 |
Take a graph paper and draw both the axes.
On the x - axis , take a scale of 1cm= 20 to represent the marks.
On the y - axis , take a scale of 1 cm =50 to represent the no. of students.
Now, plot the points (10 ,5) ,(20 ,15) ,(30 ,26) ,( 40 ,46) ,(50 ,73) ,(60 ,111), (70 ,151) ,(80 ,180) ,(90 ,194) ,(100 ,200) .
Join them by a smooth curve to get the ogive.

(i) No. of terms = 200
∴ Median = `(100 + 101)/2` = 100.5th term
Through mark of 100.5 on y-axis draw a line parallel to x-axis which meets the curve at A. From A, draw a perpendicular to x-axis which meets it at B.
The value of B is the median which is 58.5.
(ii) Lower Quartile (Q1) = `n/4 = 200/4` = 50th term
Through mark of 50 on y-axis draw a line parallel to x-axis which meets the curve at P. From P, draw a perpendicular to x-axis which meets it at Q.
The value of Q is the lower quartile which is 41 .
(iii) From marks = 80 draw a line parallel to y-axis and meet the curve at R. From R, Draw a perpendicular on y-axis which meets it at S. The difference of the value obtained when subtracted from 200 gives the number of students who scored more than 80%.
⇒ 200 - 180 = 20
20 students scored more than 80%
उत्तर २
| Less than | C.F. | Points |
| 10 | 5 | (10, 5) |
| 20 | 15 | (20, 15) |
| 30 | 26 | (30, 26) |
| 40 | 46 | (40, 46) |
| 50 | 73 | (50, 73) |
| 60 | 111 | (60, 111) |
| 70 | 151 | (70, 151) |
| 80 | 180 | (80, 180) |
| 90 | 194 | (90, 194) |
| 100 | 200 | (100, 200) |
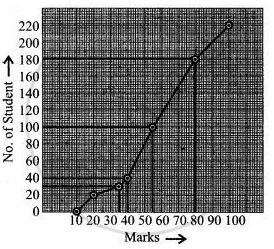
(i) Median = `"N"/(2) = (200)/(2)`
= 100 → On seeing the corresponding value.
Median = 57
(ii) Q1 = `"N"/(4) = (200)/(4)`
= 50 → On seeing the corresponding value.
= 38
(iii) 200 - 180 = 20 students
(iv) 38 students did not pass.
APPEARS IN
संबंधित प्रश्न
Calculate the mean of the distribution given below using the shortcut method.
| Marks | 11-20 | 21-30 | 31-40 | 41-50 | 51-60 | 61-70 | 71-80 |
| No. of students | 2 | 6 | 10 | 12 | 9 | 7 | 4 |
Marks obtained (in mathematics) by 9 students are given below:
60, 67, 52, 76, 50, 51, 74, 45 and 56
- Find the arithmetic mean.
- If marks of each student be increased by 4; what will be the new value of arithmetic mean?
If the mean of 6, 4, 7, ‘a’ and 10 is 8. Find the value of ‘a’
The following table gives the weekly wages of workers in a factory.
| Weekly wages (Rs) | No. of workers |
| 50 – 55 | 5 |
| 55 – 60 | 20 |
| 60 – 65 | 10 |
| 65 – 70 | 10 |
| 70 – 75 | 9 |
| 75 – 80 | 6 |
| 80 – 85 | 12 |
| 85 – 90 | 8 |
Calculate the mean by using:
Direct Method
Using step-deviation method, calculate the mean marks of the following distribution.
| C.I. | 50 – 55 | 55 – 60 | 60 – 65 | 65 – 70 | 70 – 75 | 75 – 80 | 80 – 85 | 85 – 90 |
| Frequency | 5 | 20 | 10 | 10 | 9 | 6 | 12 | 8 |
In a basket there are 10 tomatoes. The weight of each of these tomatoes in grams is as follows:
60, 70, 90, 95, 50, 65, 70, 80, 85, 95.
Find the median of the weights of tomatoes.
Find the mode of the following frequency distribution:
| Variate | 20 | 21 | 22 | 23 | 24 | 25 | 26 |
| Frequency | 21 | 20 | 26 | 35 | 22 | 13 | 10 |
Out of 10 students, who appeared in a test, three secured less than 30 marks and 3 secured more than 75 marks. The marks secured by the remaining 4 students are 35, 48, 66 and 40. Find the median score of the whole group.
Find the mean of first five prime numbers.
Find the mean of: all prime numbers upto 30
