Advertisements
Advertisements
प्रश्न
The measure of angles of a hexagon are x°, (x − 5)°, (x − 5)°, (2x − 5)°, (2x − 5)°, (2x + 20)°. Find the value of x.
उत्तर
\[\text{ Since the sum of all the angles of a hexagon is } 720° , \text{ we get } : \]
\[x° + \left( x - 5 \right)° + \left( x - 5 \right)° + \left( 2x - 5 \right)° + \left( 2x - 5 \right)° + \left( 2x + 20 \right)° = 720°\]
\[ \Rightarrow x° + x° - 5° + x°- 5°+ 2x - 5°+ 2x - 5°+ 2x + 20°= 720° \]
\[ \Rightarrow 9x - 20° + 20° = 720° \]
\[ \Rightarrow 9x = 720° \]
\[ \therefore x = 80\]
APPEARS IN
संबंधित प्रश्न
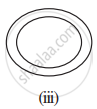
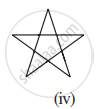
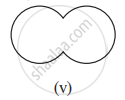
Given here are some figures:
 |
 |
 |
 |
 |
 |
 |
 |
Classify each of them on the basis of the following:
- Simple curve
- Simple closed curve
- Polygon
- Convex polygon
- Concave polygon
The three angles of a quadrilateral are respectively equal to 110°, 50° and 40°. Find its fourth angle.
A quadrilateral has three acute angles each measures 80°. What is the measure of the fourth angle?
Three angles of a quadrilateral are equal. Fourth angle is of measure 150°. What is the measure of equal angles.
The four angles of a quadrilateral are as 3 : 5 : 7 : 9. Find the angles.
The sum of the interior angles of a polygon is three times the sum of its exterior angles. Determine the number of sided of the polygon.
Two angles of a quadrilateral are 68° and 76°. If the other two angles are in the ratio 5 : 7; find the measure of each of them.
Construct a quadrilateral NEWS in which NE = 7 cm, EW = 6 cm, ∠N = 60°, ∠E = 110° and ∠S = 85°.
In given figure, What is AE + EC?
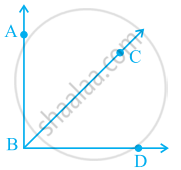
Using the information given, name the right angles in part of figure:
BA ⊥BD