Advertisements
Advertisements
рдкреНрд░рд╢реНрди
The perimeter of certain sector of circle of radius 5.6 m is 27.2 m. Find the area of sector.
рдЙрддреНрддрд░
ЁЭЬГ = angle subtended at centre
Radius (r) = 5.6m = OA ± OB
Perimeter of sector = 27.2 m
(AB arc length) + OA + OB = 27.2
⇒`(theta/360^@× 2pir)` + 5.6 + 5.6 ± 27.2
⇒`(5.6 pitheta)/180^@`+ 11.2 = 27.2
⇒ `5.6 ×22/7`× ЁЭЬГ = 16 × 180
⇒ ЁЭЬГ `=(16×180)/(0.8×22)`= 163.64°
Area of sector =`theta/360^@× pir^2 =163.64^@/360^@×22/7`× 5.6 × 5.6
=`163.64/180`× 11 × 0.8 × 5.6
= 44.8 cm2
APPEARS IN
рд╕рдВрдмрдВрдзрд┐рдд рдкреНрд░рд╢реНтАНрди
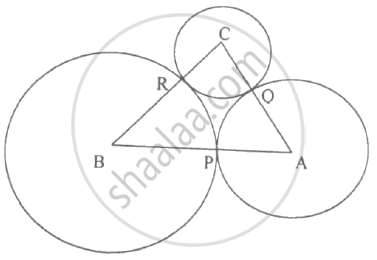
ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circles are drawn touching each other with the vertices as their centres. Find the radii of the three circles.
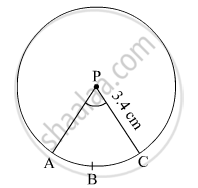
In the given figure, radius of circle is 3.4 cm and perimeter of sector P-ABC is 12.8 cm . Find A(P-ABC).
Area of a sector of central angle 200° of a circle is 770 cm2. Find the length of the corresponding arc of this sector.
A cow is tied with a rope of length 14 m at the corner of a rectangular field of dimensions 20 m × 16 m. Find the area of the field in which the cow can graze.
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
The diameter of a sphere is 6 cm, Find the total surface area of the sphere. (π = 3.14)
The circumference of a circle is 100 cm. The side of a square inscribed in the circle is ______.
Sides of a triangular field are 15 m, 16 m and 17 m. With the three corners of the field a cow, a buffalo and a horse are tied separately with ropes of length 7 m each to graze in the field. Find the area of the field which cannot be grazed by the three animals.
The length of the arc of a circle of radius 14 cm which subtends an angle of 60° at the centre of the circle is ______.
With vertices A, B and C of ΔABC as centres, arcs are drawn with radii 14 cm and the three portions of the triangle so obtained are removed. Find the total area removed from the triangle.
