Advertisements
Advertisements
प्रश्न
The present age of a father is three years more than three times the age of the son. Three years hence father's age will be 10 years more than twice the age of the son. Determine their present ages.
उत्तर
Let the present age of father be x years and the present age of his son be y years.
The present age of father is three years more than three times the age of the son. Thus, we have
`x = 3y + 3`
` ⇒ x- 3y -3 =0`
After 3 years, father’s age will be (x+3) years and son’s age will be (y + 3) years.
Thus using the given information, we have
` x + 3 = 2(y +3)+10`
` ⇒ x +3 =2y +6+10`
`⇒ x - 2y -13 =0`
So, we have two equations
`x - 3y -3 =0`
`x- 2y -13 =0`
Here x and y are unknowns. We have to solve the above equations for x and y.
By using cross-multiplication, we have
`x/((-3)xx(-13)-(-2)xx(-3))=(-y)/(1xx(-13)-1xx(-3))=1/(1xx(-2)-1xx(-3))`
`⇒ x/(39-6)=(-y)/(-13+3)=1/(-2+3)
`⇒x/33=(-y)/(-10)=1/1`
`⇒ x/33=y/10 =1`
`⇒ x= 33, y = 10`
Hence, the present age of father is 33 years and the present age of son is 10 years.
APPEARS IN
संबंधित प्रश्न
Solve the following pairs of equations by reducing them to a pair of linear equations
`4/x + 3y = 14`
`3/x - 4y = 23`
Solve the following pairs of equations by reducing them to a pair of linear equations
`1/(3x+y) + 1/(3x-y) = 3/4`
`1/(2(3x-y)) - 1/(2(3x-y)) = (-1)/8`
Solve the following pair of linear equations: px + qy = p − q, qx − py = p + q
Solve the following pair of linear equations.
(a − b) x + (a + b) y = a2− 2ab − b2
(a + b) (x + y) = a2 + b2
Solve the following pair of linear equations.
152x − 378y = − 74
− 378x + 152y = − 604
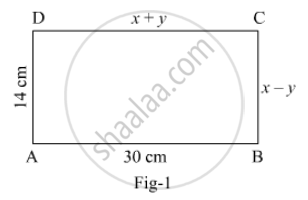
In Fig. 1, ABCD is a rectangle. Find the value of x and y.
A two-digit number is 4 times the sum of its digits. If 18 is added to the number, the digits are reversed. Find the number.
The numerator of a fraction is 4 less than the denominator. If the numerator is decreased by 2 and denominator is increased by 1, then the denominator is eight times the numerator. Find the fraction.
Let the numerator and denominator of the fraction be x and y respectively. Then the fraction is `x/y`
If the numerator is multiplied by 2 and the denominator is reduced by 5, the fraction becomes `6/5`. Thus, we have
`(2x)/(y-5)=6/5`
`⇒ 10x=6(y-5)`
`⇒ 10x=6y-30`
`⇒ 10x-6y+30 =0`
`⇒ 2(5x-3y+15)=0`
`⇒ 5x - 3y+15=0`
If the denominator is doubled and the numerator is increased by 8, the fraction becomes `2/5`. Thus, we have
`(x+8)/(2y)=2/5`
`⇒ 5(x+8)=4y`
`⇒ 5x+40=4y`
`⇒ 5x-4y+40=0`
So, we have two equations
`5x-3y+15=0`
`5x-4y+40=0`
Here x and y are unknowns. We have to solve the above equations for x and y.
By using cross-multiplication, we have
`x/((-3)xx40-(-4)xx15)=-y/(5xx40-5xx15)=1/(5xx(-4)-5xx(-3))`
`⇒ x/(-120+60)=(-y)/(200-75)=1/(-20+15)`
`⇒x/(-60)=-y/125``=1/-5`
`⇒ x= 60/5,y=125/5`
`⇒ x=12,y=25`
Hence, the fraction is `12/25`
State with reason whether the point (3, −2) will lie on the graph of the equation 5m – 3n = − 21
