Advertisements
Advertisements
प्रश्न
Solve the following pair of linear equations.
152x − 378y = − 74
− 378x + 152y = − 604
उत्तर
152x − 378y = − 74
76x − 189y = − 37
`x =(189y - 37)/76 ...(1)`
− 378x + 152y = − 604
− 189x + 76y = − 302 … (2)
Substituting the value of x in equation (2), we obtain
`-189((189y-37)/76)+76y = - 302`
− (189)2 y + 189 × 37 + (76)2 y = − 302 × 76
189 × 37 + 302 × 76 = (189)2 y − (76)2 y
6993 + 22952 = (189 − 76) (189 + 76) y
29945 = (113) (265) y
y = 1
From equation (1), we obtain
`x = (189(1)-37)/76`
`x =(189-37)/76 = 152/76`
x = 2
APPEARS IN
संबंधित प्रश्न
Solve the following simultaneous equations: `7/(2X+1)+13/(Y+2)=27,13/(2X+1)+7/(Y+2)=33`
Solve the following pairs of equations by reducing them to a pair of linear equations
`2/sqrtx +3/sqrty = 2`
`4/sqrtx - 9/sqrty = -1`
Formulate the following problems as a pair of equations, and hence find their solutions:
2 women and 5 men can together finish an embroidery work in 4 days, while 3 women and 6 men can finish it in 3 days. Find the time taken by 1 woman alone to finish the work, and also that taken by 1 man alone.
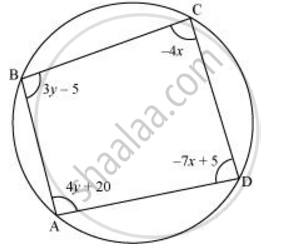
ABCD is a cyclic quadrilateral finds the angles of the cyclic quadrilateral.
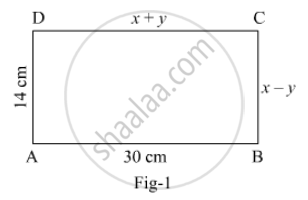
In Fig. 1, ABCD is a rectangle. Find the value of x and y.
Find the value of following determinant.
`|(5,3), (-7,0)|`
A two-digit number is 4 times the sum of its digits and twice the product of the digits. Find the number.
A two-digit number is such that the product of its digits is 20. If 9 is added to the number, the digits interchange their places. Find the number.
The present age of a father is three years more than three times the age of the son. Three years hence father's age will be 10 years more than twice the age of the son. Determine their present ages.
Two years ago, a father was five times as old as his son. Two year later, his age will be 8 more than three times the age of the son. Find the present ages of father and son.
