Question

A two-digit number is 4 times the sum of its digits and twice the product of the digits. Find the number.

Answer 1

Let the digits at units and tens place of the given number be x and y respectively. Thus, the number is `10 y + x`.

The number is 4 times the sum of the two digits. Thus, we have

` 10 y +x =4( x + y)`

` ⇒ 10y + x = 4x + 4y`

`⇒ 4x + 4y -10y -x =0 `

` ⇒ 3x -6y =0`

`⇒ 3(x - 2y)=0`

` ⇒ x- 2y =0`

` ⇒ x = 2y`

After interchanging the digits, the number becomes `10x + y`.

The number is twice the product of the digits. Thus, we have  `10y+x=2xy`

So, we have the systems of equations

` x = 2y,`

` 10y +x =2xy`

Here x and y are unknowns. We have to solve the above systems of equations for xand y.

Substituting  `x = 2y` in the second equation, we get

` 10y + 2y = 2xx2yxxy`

` ⇒ 12y = 4y^2`

` ⇒ 4y^2-12y =0`

` ⇒ y ( y -3)=0`

` ⇒ y =0` OR `y = 3`

Substituting the value of y in the first equation, we have

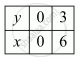
Hence, the number is `10 xx 3+6= 36.`

Note that the first pair of solution does not give a two digit number.