Advertisements
Advertisements
प्रश्न
The two similar triangles are equal in area. Prove that the triangles are congruent.
उत्तर
Given: ΔABC ∼ ΔPQR and are equal in area
To prove: ΔABC ≅ ΔPQR
Proof: ∵ ΔABC ∼ ΔPQR
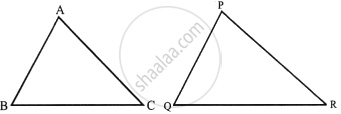
∴ `(Area ΔABC)/(Area ΔPQR) = (AB^2)/(PQ^2) = (BC^2)/(QR^2) = (AC^2)/(PR^2)`
But Area ΔABC = Area ΔPQR ...(Given)
∴ `(AB^2)/(PQ^2) = (BC^2)/(QR^2) = (AC^2)/(PR^2) = 1`
`\implies (AB^2)/(PQ^2) = 1,`
`\implies` AB2 = PQ2
`\implies` AB = PQ
Similarly, BC = QR and AC = PR
Now in ΔABC and ΔPQR
AB = PQ, BC = QR, AC = PR ...(Proved)
∴ ΔABC ≅ ΔPQR ...(SSS criterion of congruency)
APPEARS IN
संबंधित प्रश्न
In figure, QA and PB are perpendicular to AB. If AO = 10 cm, BO = 6 cm and PB = 9 cm. Find AQ
Prove that the line segments joining the mid points of the sides of a triangle form four triangles, each of which is similar to the original triangle
ΔABC~ΔPQR and ar(ΔABC) = 4, ar(ΔPQR) . If BC = 12cm, find QR.
A ship is 400m laig and 100m wide. The length of its model is 20 cm. find the surface area of the deck of the model.
In ΔABC, D and E are the mid-point on AB and AC such that DE || BC.
If AD = 8cm, AB = 12cm and AE = 12cm, find CE.
If ΔABC, D and E are points on AB and AC. Show that DE || BC for each of the following case or not:
AD = 5.7cm, BD = 9.5cm, AE = 3.3cm, and EC = 5.5cm
Through the vertex S of a parallelogram PQRS, a line is drawn to intersect the sides Qp and QR produced at M and N respectively. Prove that `"SP"/"PM" = "MQ"/"QN" = "MR"/"SR"`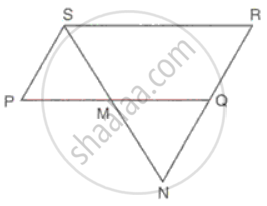
On a map drawn to a scale of 1:25000, a rectangular plot of land has sides 12cm x 16cm. Calculate: The diagonal distance of the plot in km
D is the mid point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED = x, AD = p and AE = h, prove that b2 = `"p"^2 + "a"x + "a"^2/4`
In triangle ABC point D is on side BC (B−D−C) such that ∠BAC = ∠ADC then prove that CA2 = CB × CD

