Question

Two concentric circles are of radii 5 cm and 3 cm. Length of the chord of the larger circle, (in cm), which touches the smaller circle is
(A) 4
(B) 5
(C) 8
(D) 10

Answer 1

Let O be the centre of the concentric circles.
Let AB be the chord of the larger circle touching the smaller circle at C.
Also, let OC and OB be the radii of the smaller circle and the larger circle, respectively.

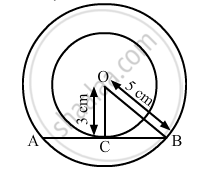
We can see that AB is the tangent to the smaller circle.
We know that the tangent at any point on a circle is perpendicular to the radius drawn to the point of contact.
∴ OC ⊥ AB

Now, in the right-angled triangle OCB:
OB² = OC² + CB² (Pythagoras theorem)
⇒ CB² = {(5)² − (3)²} cm²
           = (25 − 9) cm²
           = 16 cm²
⇒ CB = 4 cm

Now, AB = 2(CB)  (The perpendicular from the centre of the circle to the chord bisects the chord.)
⇒ AB = 8 cm
Hence, the correct option is C.