Advertisements
Advertisements
प्रश्न
Two particles A and B, each with a charge Q, are placed a distance d apart. Where should a particle of charge q be placed on the perpendicular bisector of AB, so that it experiences maximum force? What is the magnitude of this maximum force?
Advertisements
उत्तर
Let the charge q be placed at a distance x on the perpendicular bisector of AB.
As shown in the figure, the horizontal component of force is balanced.
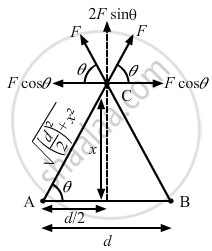
\[\sin\theta = \frac{x}{\sqrt{\left( \frac{d}{2} \right)^2 + x^2}}\]
Total vertical component of force,
\[F' = 2F\sin\theta\]
\[F' = 2 \times \frac{1}{4\pi \epsilon_0} \times \frac{qQ}{\left( \frac{d}{2} \right)^2 + x^2} \times \frac{x}{\sqrt{\left( \frac{d}{2} \right)^2 + x^2}}\]
\[ \Rightarrow F' = \frac{1}{2\pi \epsilon_0} \times \frac{qQx}{\left[ \left( \frac{d}{2} \right)^2 + x^2 \right]^{3/2}}\]
For maximum force, \[\frac{dF'}{dx} = 0\]
\[\frac{qQ}{2\pi \epsilon_0} \times \left[ \left[ \left( \frac{d}{2} \right)^2 + x^2 \right]^{- 3/2} - x\frac{3}{2} \left[ \left( \frac{d}{2} \right)^2 + x^2 \right]^{- 5/2} 2x \right] = 0\]
\[ \Rightarrow \left( \frac{d}{2} \right)^2 + x^2 - 3 x^2 = 0\]
\[ \Rightarrow 2 x^2 = \left( \frac{d}{2} \right)^2 \]
\[ \Rightarrow x = \frac{d}{2\sqrt{2}}\]
\[ \therefore F '_\max = \frac{1}{2\pi \epsilon_0}\frac{qQ\frac{d}{2\sqrt{2}}}{\left[ \left( \frac{d}{2} \right)^2 + \left( \frac{d}{2\sqrt{2}} \right)^2 \right]^{3/2}}\]
\[ = 3 . 08\frac{Qq}{4\pi \epsilon_0 d^2}\]
