Advertisements
Advertisements
प्रश्न
Two vectors have magnitudes 2 unit and 4 unit respectively. What should be the angle between them if the magnitude of the resultant is (a) 1 unit, (b) 5 unit and (c) 7 unit.
उत्तर
Let the two vectors be \[\vec{a}\] and \[\vec{b}\]
Now,
\[\left| \vec{a} \right| = 3 \text { and } \left| \vec{b} \right| = 4\]
\[\sqrt{\vec{a}^2 + \vec{b}^2 + 2 . \vec{a} . \vec{b} \cos \theta} = 1\]
\[ \Rightarrow \sqrt{3^2 + 4^2 + 2 . 3 . 4 \cos \theta} = 1\]
Squaring both sides, we get:
\[25 + 24 \cos \theta = 1\]
\[ \Rightarrow 24 \cos \theta = - 24\]
\[ \Rightarrow \cos \theta = - 1\]
\[ \Rightarrow \theta = 180^\circ\]
Hence, the angle between them is 180°.
(b) If the resultant vector is 5 units, then
\[\sqrt{\vec{a}^2 + \vec{b}^2 + 2 . \vec{a} . \vec{b} \cos \theta} = 5\]
\[ \Rightarrow \sqrt{3^2 + 4^2 + 2 . 3 . 4 \cos \theta} = 5\]
Squaring both sides, we get:
25 + 24 cos θ = 25
⇒ 24 cos θ = 0
⇒ cos θ = 90°
Hence, the angle between them is 90°.
(c) If the resultant vector is 7 units, then
\[\sqrt{\vec{a}^2 + \vec{b}^2 + 2 . \vec{a} . \vec{b} \cos \theta} = 1\]
\[ \Rightarrow \sqrt{3^2 + 4^2 + 2 . 3 . 4 \cos \theta} = 7\]
Squaring both sides, we get:
25 + 24 cos θ = 49,
⇒ 24 cos θ = 24
⇒ cos θ = 1
⇒ θ = cos−1 1 = 0°
Hence, the angle between them is 0°.
APPEARS IN
संबंधित प्रश्न
Some of the most profound statements on the nature of science have come from Albert Einstein, one of the greatest scientists of all time. What do you think did Einstein mean when he said : “The most incomprehensible thing about the world is that it is comprehensible”?
What are the dimensions of the ratio of the volume of a cube of edge a to the volume of a sphere of radius a?
If all the terms in an equation have same units, is it necessary that they have same dimensions? If all the terms in an equation have same dimensions, is it necessary that they have same units?
If two quantities have same dimensions, do they represent same physical content?
It is desirable that the standards of units be easily available, invariable, indestructible and easily reproducible. If we use foot of a person as a standard unit of length, which of the above features are present and which are not?
A unitless quantity
Choose the correct statements(s):
(a) All quantities may be represented dimensionally in terms of the base quantities.
(b) A base quantity cannot be represented dimensionally in terms of the rest of the base quantities.
(c) The dimensions of a base quantity in other base quantities is always zero.
(d) The dimension of a derived quantity is never zero in any base quantity.
Find the dimensions of the coefficient of linear expansion α and
Is the vector sum of the unit vectors \[\vec{i}\] and \[\vec{i}\] a unit vector? If no, can you multiply this sum by a scalar number to get a unit vector?
The component of a vector is
The radius of a circle is stated as 2.12 cm. Its area should be written as
Let \[\vec{C} = \vec{A} + \vec{B}\]
Let the angle between two nonzero vectors \[\vec{A}\] and \[\vec{B}\] be 120° and its resultant be \[\vec{C}\].
Let \[\vec{A} \text { and } \vec{B}\] be the two vectors of magnitude 10 unit each. If they are inclined to the X-axis at angle 30° and 60° respectively, find the resultant.
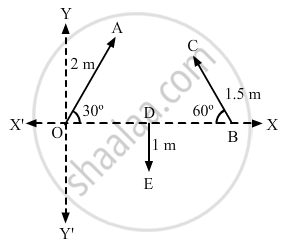
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
A mosquito net over a 7 ft × 4 ft bed is 3 ft high. The net has a hole at one corner of the bed through which a mosquito enters the net. It flies and sits at the diagonally opposite upper corner of the net. (a) Find the magnitude of the displacement of the mosquito. (b) Taking the hole as the origin, the length of the bed as the X-axis, it width as the Y axis, and vertically up as the Z-axis, write the components of the displacement vector.
If \[\vec{A} , \vec{B} , \vec{C}\] are mutually perpendicular, show that \[\vec{C} \times \left( \vec{A} \times \vec{B} \right) = 0\] Is the converse true?
Give an example for which \[\vec{A} \cdot \vec{B} = \vec{C} \cdot \vec{B} \text{ but } \vec{A} \neq \vec{C}\].
The changes in a function y and the independent variable x are related as
\[\frac{dy}{dx} = x^2\] . Find y as a function of x.
