Advertisements
Advertisements
प्रश्न
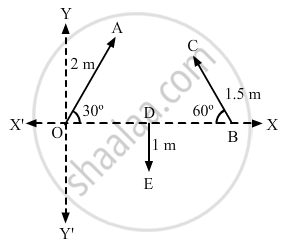
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
उत्तर
First, let us find the components of the vectors along the x and y-axes. Then we will find the resultant x and y-components.
x-component of \[\overrightarrow{OA} = 2 \cos 30^\circ= \sqrt{3}\]
x-component of \[\overrightarrow{BC}\] = 1.5 cos120°
\[= - \frac{\left( 1 . 5 \right)}{2} = - 7 . 5\]
x-component of \[\overrightarrow{DE}\] = 1 cos 270°
= 1 × 0 = 0 m
y-component of \[\overrightarrow {OA}\] = 2 sin 30° = 1
y-component of \[\overrightarrow{BC}\] = 1.5 sin 120°
\[= \frac{\left( \sqrt{3} \times 1 . 5 \right)}{2} = 1 . 3\]
y-component of \[\overrightarrow{DE}\] = 1 sin 270° = −1
x-component of resultant \[R_x = \sqrt{3} - 0 . 75 + 0 = 0 . 98 m\]
y-component of resultant Ry = 1 + 1.3 − 1 = 1.3 m
\[\therefore \text { Resultant, R }= \sqrt{\left( R_x \right)^2 + \left( R_y \right)^2}\]
\[ = \sqrt{\left( 0 . 98 \right)^2 + \left( 1 . 3 \right)^2}\]
\[ = \sqrt{0 . 96 + 1 . 69}\]
\[ = \sqrt{2 . 65}\]
\[ = 1 . 6 m\]
If it makes an angle α with the positive x-axis, then
\[\tan \alpha = \frac{\text { y -component }}{\text { x - component }}\]
\[ = \frac{1 . 3}{0 . 98} = 1 . 332\]
APPEARS IN
संबंधित प्रश्न
Some of the most profound statements on the nature of science have come from Albert Einstein, one of the greatest scientists of all time. What do you think did Einstein mean when he said : “The most incomprehensible thing about the world is that it is comprehensible”?
“It is more important to have beauty in the equations of physics than to have them agree with experiments”. The great British physicist P. A. M. Dirac held this view. Criticize this statement. Look out for some equations and results in this book which strike you as beautiful.
If two quantities have same dimensions, do they represent same physical content?
A unitless quantity
\[\int\frac{dx}{\sqrt{2ax - x^2}} = a^n \sin^{- 1} \left[ \frac{x}{a} - 1 \right]\]
The value of n is
Find the dimensions of linear momentum .
Find the dimensions of pressure.
Find the dimensions of electric field E.
The relevant equations are \[F = qE, F = qvB, \text{ and }B = \frac{\mu_0 I}{2 \pi a};\]
where F is force, q is charge, v is speed, I is current, and a is distance.
Can you add three unit vectors to get a unit vector? Does your answer change if two unit vectors are along the coordinate axes?
Let ε1 and ε2 be the angles made by \[\vec{A}\] and -\[\vec{A}\] with the positive X-axis. Show that tan ε1 = tan ε2. Thus, giving tan ε does not uniquely determine the direction of \[\vec{A}\].
Is the vector sum of the unit vectors \[\vec{i}\] and \[\vec{i}\] a unit vector? If no, can you multiply this sum by a scalar number to get a unit vector?
Can you have \[\vec{A} \times \vec{B} = \vec{A} \cdot \vec{B}\] with A ≠ 0 and B ≠ 0 ? What if one of the two vectors is zero?
A situation may be described by using different sets coordinate axes having different orientation. Which the following do not depended on the orientation of the axis?
(a) the value of a scalar
(b) component of a vector
(c) a vector
(d) the magnitude of a vector.
The x-component of the resultant of several vectors
(a) is equal to the sum of the x-components of the vectors of the vectors
(b) may be smaller than the sum of the magnitudes of the vectors
(c) may be greater than the sum of the magnitudes of the vectors
(d) may be equal to the sum of the magnitudes of the vectors.
Add vectors \[\vec{A} , \vec{B} \text { and } \vec{C}\] each having magnitude of 100 unit and inclined to the X-axis at angles 45°, 135° and 315° respectively.
A spy report about a suspected car reads as follows. "The car moved 2.00 km towards east, made a perpendicular left turn, ran for 500 m, made a perpendicular right turn, ran for 4.00 km and stopped". Find the displacement of the car.
Let A1 A2 A3 A4 A5 A6 A1 be a regular hexagon. Write the x-components of the vectors represented by the six sides taken in order. Use the fact the resultant of these six vectors is zero, to prove that
cos 0 + cos π/3 + cos 2π/3 + cos 3π/3 + cos 4π/3 + cos 5π/3 = 0.
Use the known cosine values to verify the result.

Let \[\vec{a} = 2 \vec{i} + 3 \vec{j} + 4 \vec{k} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j} + 5 \vec{k}\] Find the angle between them.
Jupiter is at a distance of 824.7 million km from the Earth. Its angular diameter is measured to be 35.72˝. Calculate the diameter of Jupiter.
