Advertisements
Advertisements
Question
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
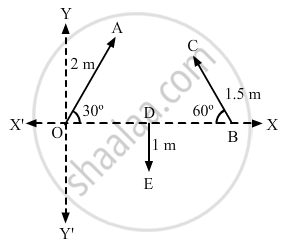
Solution
First, let us find the components of the vectors along the x and y-axes. Then we will find the resultant x and y-components.
x-component of \[\overrightarrow{OA} = 2 \cos 30^\circ= \sqrt{3}\]
x-component of \[\overrightarrow{BC}\] = 1.5 cos120°
\[= - \frac{\left( 1 . 5 \right)}{2} = - 7 . 5\]
x-component of \[\overrightarrow{DE}\] = 1 cos 270°
= 1 × 0 = 0 m
y-component of \[\overrightarrow {OA}\] = 2 sin 30° = 1
y-component of \[\overrightarrow{BC}\] = 1.5 sin 120°
\[= \frac{\left( \sqrt{3} \times 1 . 5 \right)}{2} = 1 . 3\]
y-component of \[\overrightarrow{DE}\] = 1 sin 270° = −1
x-component of resultant \[R_x = \sqrt{3} - 0 . 75 + 0 = 0 . 98 m\]
y-component of resultant Ry = 1 + 1.3 − 1 = 1.3 m
\[\therefore \text { Resultant, R }= \sqrt{\left( R_x \right)^2 + \left( R_y \right)^2}\]
\[ = \sqrt{\left( 0 . 98 \right)^2 + \left( 1 . 3 \right)^2}\]
\[ = \sqrt{0 . 96 + 1 . 69}\]
\[ = \sqrt{2 . 65}\]
\[ = 1 . 6 m\]
If it makes an angle α with the positive x-axis, then
\[\tan \alpha = \frac{\text { y -component }}{\text { x - component }}\]
\[ = \frac{1 . 3}{0 . 98} = 1 . 332\]
APPEARS IN
RELATED QUESTIONS
What are the dimensions of volume of a sphere of radius a?
What are the dimensions of the ratio of the volume of a cube of edge a to the volume of a sphere of radius a?
A physical quantity is measured and the result is expressed as nu where u is the unit used and n is the numerical value. If the result is expressed in various units then
A dimensionless quantity
The dimensions ML−1 T−2 may correspond to
Choose the correct statements(s):
Find the dimensions of frequency .
Find the dimensions of
(a) angular speed ω,
(b) angular acceleration α,
(c) torque τ and
(d) moment of interia I.
Some of the equations involving these quantities are \[\omega = \frac{\theta_2 - \theta_1}{t_2 - t_1}, \alpha = \frac{\omega_2 - \omega_1}{t_2 - t_1}, \tau = F . r \text{ and }I = m r^2\].
The symbols have standard meanings.
Find the dimensions of the specific heat capacity c.
(a) the specific heat capacity c,
(b) the coefficient of linear expansion α and
(c) the gas constant R.
Some of the equations involving these quantities are \[Q = mc\left( T_2 - T_1 \right), l_t = l_0 \left[ 1 + \alpha\left( T_2 - T_1 \right) \right]\] and PV = nRT.
The height of mercury column in a barometer in a Calcutta laboratory was recorded to be 75 cm. Calculate this pressure in SI and CGS units using the following data : Specific gravity of mercury = \[13 \cdot 6\] , Density of \[\text{ water} = {10}^3 kg/ m^3 , g = 9 \cdot 8 m/ s^2\] at Calcutta. Pressure
= hpg in usual symbols.
Is the vector sum of the unit vectors \[\vec{i}\] and \[\vec{i}\] a unit vector? If no, can you multiply this sum by a scalar number to get a unit vector?
If \[\vec{A} \times \vec{B} = 0\] can you say that
(a) \[\vec{A} = \vec{B} ,\]
(b) \[\vec{A} \neq \vec{B}\] ?
A vector is not changed if
The x-component of the resultant of several vectors
(a) is equal to the sum of the x-components of the vectors of the vectors
(b) may be smaller than the sum of the magnitudes of the vectors
(c) may be greater than the sum of the magnitudes of the vectors
(d) may be equal to the sum of the magnitudes of the vectors.
A vector \[\vec{A}\] makes an angle of 20° and \[\vec{B}\] makes an angle of 110° with the X-axis. The magnitudes of these vectors are 3 m and 4 m respectively. Find the resultant.
A carrom board (4 ft × 4 ft square) has the queen at the centre. The queen, hit by the striker moves to the from edge, rebounds and goes in the hole behind the striking line. Find the magnitude of displacement of the queen (a) from the centre to the front edge, (b) from the front edge to the hole and (c) from the centre to the hole.
Give an example for which \[\vec{A} \cdot \vec{B} = \vec{C} \cdot \vec{B} \text{ but } \vec{A} \neq \vec{C}\].
High speed moving particles are studied under
