Advertisements
Online Mock Tests
Chapters
▶ 2: Physics and Mathematics
3: Rest and Motion: Kinematics
4: The Forces
5: Newton's Laws of Motion
6: Friction
7: Circular Motion
8: Work and Energy
9: Centre of Mass, Linear Momentum, Collision
10: Rotational Mechanics
11: Gravitation
12: Simple Harmonics Motion
13: Fluid Mechanics
14: Some Mechanical Properties of Matter
15: Wave Motion and Waves on a String
16: Sound Waves
17: Light Waves
18: Geometrical Optics
19: Optical Instruments
20: Dispersion and Spectra
21: Speed of Light
22: Photometry
![HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 chapter 2 - Physics and Mathematics HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 chapter 2 - Physics and Mathematics - Shaalaa.com](/images/9788177091878-concepts-of-physics-vol-1-english-class-11-and-12_6:46896509a13743528b4d5729ac982f47.jpg)
Advertisements
Solutions for Chapter 2: Physics and Mathematics
Below listed, you can find solutions for Chapter 2 of CBSE, Karnataka Board PUC HC Verma for Concepts of Physics Vol. 1 [English] Class 11 and 12.
HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 2 Physics and Mathematics Short Answers [Pages 27 - 28]
Is a vector necessarily changed if it is rotated through an angle?
Is it possible to add two vectors of unequal magnitudes and get zero? Is it possible to add three vectors of equal magnitudes and get zero?
Does the phrase "direction of zero vector" have physical significance? Discuss it terms of velocity, force etc.
Can you add three unit vectors to get a unit vector? Does your answer change if two unit vectors are along the coordinate axes?
Can we have physical quantities having magnitude and direction which are not vectors?
Which of the following two statements is more appropriate?
(a) Two forces are added using the triangle rule because force is a vector quantity.
(b) Force is a vector quantity because two forces are added using the triangle rule.
Can you add two vectors representing physical quantities having different dimensions? Can you multiply two vectors representing physical quantities having different dimensions?
Can a vector have zero component along a line and still have nonzero magnitude?
Let ε1 and ε2 be the angles made by \[\vec{A}\] and -\[\vec{A}\] with the positive X-axis. Show that tan ε1 = tan ε2. Thus, giving tan ε does not uniquely determine the direction of \[\vec{A}\].
Is the vector sum of the unit vectors \[\vec{i}\] and \[\vec{i}\] a unit vector? If no, can you multiply this sum by a scalar number to get a unit vector?
Let \[\vec{A} = 3 \vec{i} + 4 \vec{j}\]. Write a vector \[\vec{B}\] such that \[\vec{A} \neq \vec{B}\], but A = B.
Can you have \[\vec{A} \times \vec{B} = \vec{A} \cdot \vec{B}\] with A ≠ 0 and B ≠ 0 ? What if one of the two vectors is zero?
If \[\vec{A} \times \vec{B} = 0\] can you say that
(a) \[\vec{A} = \vec{B} ,\]
(b) \[\vec{A} \neq \vec{B}\] ?
Let \[\vec{A} = 5 \vec{i} - 4 \vec{j} \text { and } \vec{B} = - 7 \cdot 5 \vec{i} + 6 \vec{j}\]. Do we have \[\vec{B} = k \vec{A}\] ? Can we say \[\frac{\vec{B}}{\vec{A}}\] = k ?
HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 2 Physics and Mathematics MCQ [Page 28]
A vector is not changed if
it is rotated through an arbitrary angle
it is multiplied by an arbitrary scalar
it is cross multiplied by a unit vector
it is slid parallel to itself.
Which of the sets given below may represent the magnitudes of three vectors adding to zero?
2, 4, 8
4, 8, 16
1, 2, 1
0.5, 1, 2
The resultant of \[\vec{A} \text { and } \vec{B}\] makes an angle α with \[\vec{A}\] and β with \[\vec{B}\],
α < β
α < β if A < B
α < β if A > B
α < β if A = B
The component of a vector is
always less than its magnitude
always greater than its magnitude
always equal to its magnitude
None of these.
A vector \[\vec{A}\] points vertically upward and \[\vec{B}\] points towards the north. The vector product \[\vec{A} \times \vec{B}\] is
along the west
along the east
zero
vertically downward.
The radius of a circle is stated as 2.12 cm. Its area should be written as
14 cm2
14.1 cm2
14.11 cm2
14.1124 cm2
HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 2 Physics and Mathematics MCQ [Page 28]
A situation may be described by using different sets coordinate axes having different orientation. Which the following do not depended on the orientation of the axis?
(a) the value of a scalar
(b) component of a vector
(c) a vector
(d) the magnitude of a vector.
Let \[\vec{C} = \vec{A} + \vec{B}\]
\[\left| \vec{C} \right|\] is always greater than \[\left| \vec{A} \right|\]
It is possible to have \[\left| \vec{C} \right|\] < \[\left| \vec{A} \right|\] and \[\left| \vec{C} \right|\] < \[\left| \vec{B} \right|\]
C is always equal to A + B
C is never equal to A + B.
Let the angle between two nonzero vectors \[\vec{A}\] and \[\vec{B}\] be 120° and its resultant be \[\vec{C}\].
C must be equal to \[\left| A - B \right|\]
C must be less than \[\left| A - B \right|\]
C must be greater than \[\left| A - B \right|\]
C may be equal to \[\left| A - B \right|\]
The x-component of the resultant of several vectors
(a) is equal to the sum of the x-components of the vectors of the vectors
(b) may be smaller than the sum of the magnitudes of the vectors
(c) may be greater than the sum of the magnitudes of the vectors
(d) may be equal to the sum of the magnitudes of the vectors.
The magnitude of the vector product of two vectors \[\left| \vec{A} \right|\] and \[\left| \vec{B} \right|\] may be
(a) greater than AB
(b) equal to AB
(c) less than AB
(d) equal to zero.
HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 2 Physics and Mathematics Exercise [Pages 29 - 30]
A vector \[\vec{A}\] makes an angle of 20° and \[\vec{B}\] makes an angle of 110° with the X-axis. The magnitudes of these vectors are 3 m and 4 m respectively. Find the resultant.
Let \[\vec{A} \text { and } \vec{B}\] be the two vectors of magnitude 10 unit each. If they are inclined to the X-axis at angle 30° and 60° respectively, find the resultant.
Add vectors \[\vec{A} , \vec{B} \text { and } \vec{C}\] each having magnitude of 100 unit and inclined to the X-axis at angles 45°, 135° and 315° respectively.
Let \[\vec{a} = 4 \vec{i} + 3 \vec{j} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j}\]. Find the magnitudes of (a) \[\vec{a}\] , (b) \[\vec{b}\] ,(c) \[\vec{a} + \vec{b} \text { and }\] (d) \[\vec{a} - \vec{b}\].
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
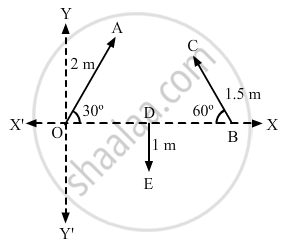
Two vectors have magnitudes 2 unit and 4 unit respectively. What should be the angle between them if the magnitude of the resultant is (a) 1 unit, (b) 5 unit and (c) 7 unit.
A spy report about a suspected car reads as follows. "The car moved 2.00 km towards east, made a perpendicular left turn, ran for 500 m, made a perpendicular right turn, ran for 4.00 km and stopped". Find the displacement of the car.
A carrom board (4 ft × 4 ft square) has the queen at the centre. The queen, hit by the striker moves to the from edge, rebounds and goes in the hole behind the striking line. Find the magnitude of displacement of the queen (a) from the centre to the front edge, (b) from the front edge to the hole and (c) from the centre to the hole.
A mosquito net over a 7 ft × 4 ft bed is 3 ft high. The net has a hole at one corner of the bed through which a mosquito enters the net. It flies and sits at the diagonally opposite upper corner of the net. (a) Find the magnitude of the displacement of the mosquito. (b) Taking the hole as the origin, the length of the bed as the X-axis, it width as the Y axis, and vertically up as the Z-axis, write the components of the displacement vector.
Suppose \[\vec{a}\] is a vector of magnitude 4.5 units due north. What is the vector (a) \[3 \vec{a}\], (b) \[- 4 \vec{a}\] ?
Two vectors have magnitudes 2 m and 3m. The angle between them is 60°. Find (a) the scalar product of the two vectors, (b) the magnitude of their vector product.
Let A1 A2 A3 A4 A5 A6 A1 be a regular hexagon. Write the x-components of the vectors represented by the six sides taken in order. Use the fact the resultant of these six vectors is zero, to prove that
cos 0 + cos π/3 + cos 2π/3 + cos 3π/3 + cos 4π/3 + cos 5π/3 = 0.
Use the known cosine values to verify the result.

Let \[\vec{a} = 2 \vec{i} + 3 \vec{j} + 4 \vec{k} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j} + 5 \vec{k}\] Find the angle between them.
Prove that \[\vec{A} . \left( \vec{A} \times \vec{B} \right) = 0\].
If \[\vec{A} = 2 \vec{i} + 3 \vec{j} + 4 \vec{k} \text { and } \vec{B} = 4 \vec{i} + 3 \vec{j} + 2 \vec{k}\] find \[\vec{A} \times \vec{B}\].
If \[\vec{A} , \vec{B} , \vec{C}\] are mutually perpendicular, show that \[\vec{C} \times \left( \vec{A} \times \vec{B} \right) = 0\] Is the converse true?
A particle moves on a given straight line with a constant speed ν. At a certain time it is at a point P on its straight line path. O is a fixed point. Show that \[\vec{OP} \times \vec{\nu}\] is independent of the position P.
The force on a charged particle due to electric and magnetic fields is given by \[\vec{F} = q \vec{E} + q \vec{\nu} \times \vec{B}\].
Suppose \[\vec{E}\] is along the X-axis and \[\vec{B}\] along the Y-axis. In what direction and with what minimum speed ν should a positively charged particle be sent so that the net force on it is zero?
Give an example for which \[\vec{A} \cdot \vec{B} = \vec{C} \cdot \vec{B} \text{ but } \vec{A} \neq \vec{C}\].
Draw a graph from the following data. Draw tangents at x = 2, 4, 6 and 8. Find the slopes of these tangents. Verify that the curve draw is y = 2x2 and the slope of tangent is \[\tan \theta = \frac{dy}{dx} = 4x\]
\[\begin{array}x & 1 & 2 & 3 & 4 & 5 & 6 & 7 & 8 & 9 & 10 \\ y & 2 & 8 & 18 & 32 & 50 & 72 & 98 & 128 & 162 & 200\end{array}\]
A curve is represented by y = sin x. If x is changed from \[\frac{\pi}{3}\text{ to }\frac{\pi}{3} + \frac{\pi}{100}\] , find approximately the change in y.
The electric current in a charging R−C circuit is given by i = i0 e−t/RC where i0, R and C are constant parameters of the circuit and t is time. Find the rate of change of current at (a) t = 0, (b) t = RC, (c) t = 10 RC.
The electric current in a discharging R−C circuit is given by i = i0 e−t/RC where i0, R and C are constant parameters and t is time. Let i0 = 2⋅00 A, R = 6⋅00 × 105 Ω and C = 0⋅500 μF. (a) Find the current at t = 0⋅3 s. (b) Find the rate of change of current at at 0⋅3 s. (c) Find approximately the current at t = 0⋅31 s.
Find the area bounded under the curve y = 3x2 + 6x + 7 and the X-axis with the ordinates at x = 5 and x = 10.
Find the area enclosed by the curve y = sin x and the X-axis between x = 0 and x = π.
Find the area bounded by the curve y = e−x, the X-axis and the Y-axis.
A rod of length L is placed along the X-axis between x = 0 and x = L. The linear density (mass/length) ρ of the rod varies with the distance x from the origin as ρ = a + bx. (a) Find the SI units of a and b. (b) Find the mass of the rod in terms of a, b and L.
The momentum p of a particle changes with time t according to the relation
\[\frac{dp}{dt} = \left( 10 N \right) + \left( 2 N/s \right)t\] If the momentum is zero at t = 0, what will the momentum be at t = 10 s?
The changes in a function y and the independent variable x are related as
\[\frac{dy}{dx} = x^2\] . Find y as a function of x.
Write the number of significant digits in (a) 1001, (b) 100.1, (c) 100.10, (d) 0.001001.
A metre scale is graduated at every millimetre. How many significant digits will be there in a length measurement with this scale?
Round the following numbers to 2 significant digits.
(a) 3472, (b) 84.16. (c)2.55 and (d) 28.5
The length and the radius of a cylinder measured with a slide callipers are found to be 4.54 cm and 1.75 cm respectively. Calculate the volume of the cylinder.
The thickness of a glass plate is measured to be 2.17 mm, 2.17 mm and 2.18 mm at three different places. Find the average thickness of the plate from this data.
The length of the string of a simple pendulum is measured with a metre scale to be 90.0 cm. The radius of the bod plus the length of the hook is calculated to be 2.13 cm using measurements with a slide callipers. What is the effective length of the pendulum? (The effective length is defined as the distance between the point of suspension and the centre of the bob.)
Solutions for 2: Physics and Mathematics
![HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 chapter 2 - Physics and Mathematics HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 chapter 2 - Physics and Mathematics - Shaalaa.com](/images/9788177091878-concepts-of-physics-vol-1-english-class-11-and-12_6:46896509a13743528b4d5729ac982f47.jpg)
HC Verma solutions for Concepts of Physics Vol. 1 [English] Class 11 and 12 chapter 2 - Physics and Mathematics
Shaalaa.com has the CBSE, Karnataka Board PUC Mathematics Concepts of Physics Vol. 1 [English] Class 11 and 12 CBSE, Karnataka Board PUC solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. HC Verma solutions for Mathematics Concepts of Physics Vol. 1 [English] Class 11 and 12 CBSE, Karnataka Board PUC 2 (Physics and Mathematics) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. HC Verma textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Concepts of Physics Vol. 1 [English] Class 11 and 12 chapter 2 Physics and Mathematics are Physics Related to Technology and Society, What is Physics?, Scope and Excitement of Physics, Nature of Physical Laws, What is Science?, The Scientific Method, Branches of Physics, Fundamental Forces in Nature.
Using HC Verma Concepts of Physics Vol. 1 [English] Class 11 and 12 solutions Physics and Mathematics exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in HC Verma Solutions are essential questions that can be asked in the final exam. Maximum CBSE, Karnataka Board PUC Concepts of Physics Vol. 1 [English] Class 11 and 12 students prefer HC Verma Textbook Solutions to score more in exams.
Get the free view of Chapter 2, Physics and Mathematics Concepts of Physics Vol. 1 [English] Class 11 and 12 additional questions for Mathematics Concepts of Physics Vol. 1 [English] Class 11 and 12 CBSE, Karnataka Board PUC, and you can use Shaalaa.com to keep it handy for your exam preparation.
