Advertisements
Advertisements
Question
The changes in a function y and the independent variable x are related as
\[\frac{dy}{dx} = x^2\] . Find y as a function of x.
Solution
Changes in a function of y and the independent variable x are related as follows:
\[\frac{dy}{dx} = x^2 \]
\[ \Rightarrow dy = x^2 dx\]
Integrating of both sides, we get:
∫dy = ∫x2 dx
\[\Rightarrow y = \frac{x^3}{3} + c\]
where c is a constant
∴ y as a function of x is represented by
\[y = \frac{x^3}{3} + c\]
APPEARS IN
RELATED QUESTIONS
“Every great physical theory starts as a heresy and ends as a dogma”. Give some examples from the history of science of the validity of this incisive remark
What are the dimensions of volume of a sphere of radius a?
If all the terms in an equation have same units, is it necessary that they have same dimensions? If all the terms in an equation have same dimensions, is it necessary that they have same units?
Suggest a way to measure the thickness of a sheet of paper.
Find the dimensions of linear momentum .
Find the dimensions of the specific heat capacity c.
(a) the specific heat capacity c,
(b) the coefficient of linear expansion α and
(c) the gas constant R.
Some of the equations involving these quantities are \[Q = mc\left( T_2 - T_1 \right), l_t = l_0 \left[ 1 + \alpha\left( T_2 - T_1 \right) \right]\] and PV = nRT.
Can you add two vectors representing physical quantities having different dimensions? Can you multiply two vectors representing physical quantities having different dimensions?
Is the vector sum of the unit vectors \[\vec{i}\] and \[\vec{i}\] a unit vector? If no, can you multiply this sum by a scalar number to get a unit vector?
Let \[\vec{A} = 3 \vec{i} + 4 \vec{j}\]. Write a vector \[\vec{B}\] such that \[\vec{A} \neq \vec{B}\], but A = B.
Let \[\vec{A} = 5 \vec{i} - 4 \vec{j} \text { and } \vec{B} = - 7 \cdot 5 \vec{i} + 6 \vec{j}\]. Do we have \[\vec{B} = k \vec{A}\] ? Can we say \[\frac{\vec{B}}{\vec{A}}\] = k ?
The component of a vector is
Let \[\vec{C} = \vec{A} + \vec{B}\]
Let \[\vec{a} = 4 \vec{i} + 3 \vec{j} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j}\]. Find the magnitudes of (a) \[\vec{a}\] , (b) \[\vec{b}\] ,(c) \[\vec{a} + \vec{b} \text { and }\] (d) \[\vec{a} - \vec{b}\].
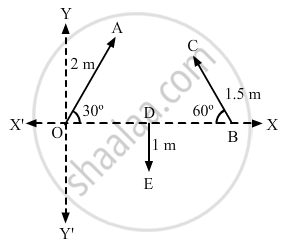
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
Two vectors have magnitudes 2 m and 3m. The angle between them is 60°. Find (a) the scalar product of the two vectors, (b) the magnitude of their vector product.
Let \[\vec{a} = 2 \vec{i} + 3 \vec{j} + 4 \vec{k} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j} + 5 \vec{k}\] Find the angle between them.
Give an example for which \[\vec{A} \cdot \vec{B} = \vec{C} \cdot \vec{B} \text{ but } \vec{A} \neq \vec{C}\].
Jupiter is at a distance of 824.7 million km from the Earth. Its angular diameter is measured to be 35.72˝. Calculate the diameter of Jupiter.
If π = 3.14, then the value of π2 is ______
