Advertisements
Advertisements
Question
A carrom board (4 ft × 4 ft square) has the queen at the centre. The queen, hit by the striker moves to the from edge, rebounds and goes in the hole behind the striking line. Find the magnitude of displacement of the queen (a) from the centre to the front edge, (b) from the front edge to the hole and (c) from the centre to the hole.
Solution
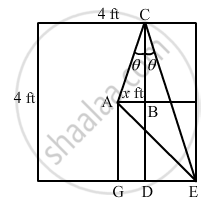
Consider that the queen is initially at point A as shown in the figure.
Let AB be x ft.
So, DE = (2 \[-\] x) ft
In ∆ABC, we have: \[\tan \theta = \frac{x}{2}\] ...(i)
Also, in ∆DCE, we have:
\[\tan \theta = \frac{\left( 2 - x \right)}{4}\] ...(ii)
From (i) and (ii), we get:
\[\frac{x}{2} = \frac{\left( 2 - x \right)}{4}\]
\[ \Rightarrow 2\left( 2 - x \right) = 4x\]
\[ \Rightarrow 4 - 2x = 4x\]
\[ \Rightarrow 6x = 4\]
\[ \Rightarrow x = \frac{2}{3}\text { ft }\]
(a) In ∆ABC, we have:
\[AC = \sqrt{{AB}^2 + {BC}^2}\]
\[= \sqrt{\left( \frac{2}{3} \right)^2 + 2^2}\]
\[ = \sqrt{\frac{4}{9} + 4} = \sqrt{\frac{40}{9}}\]
\[ = \frac{2}{3}\sqrt{10} \text { ft }\]
(b) In ∆CDE, we have:
DE \[= 2 - \frac{2}{3} = \frac{6 - 2}{3} = \frac{4}{3} \text { ft }\]
CD = 4 ft
\[\therefore CE = \sqrt{{CD}^2 + {DE}^2}\]
\[ = \sqrt{4^2 + \left( \frac{4}{3} \right)^2}\]
\[ = \frac{4}{3}\sqrt{10} \text { ft }\]
(c) In ∆AGE, we have:
\[AE = \sqrt{{AG}^2 + {GE}^2}\]
\[= \sqrt{2^2 + 2^2}\]
\[\sqrt{8} = 2\sqrt{2}\text { ft }\]
APPEARS IN
RELATED QUESTIONS
“Every great physical theory starts as a heresy and ends as a dogma”. Give some examples from the history of science of the validity of this incisive remark
What are the dimensions of volume of a sphere of radius a?
A physical quantity is measured and the result is expressed as nu where u is the unit used and n is the numerical value. If the result is expressed in various units then
Suppose a quantity x can be dimensionally represented in terms of M, L and T, that is, `[ x ] = M^a L^b T^c`. The quantity mass
A dimensionless quantity
Find the dimensions of frequency .
Find the dimensions of the specific heat capacity c.
(a) the specific heat capacity c,
(b) the coefficient of linear expansion α and
(c) the gas constant R.
Some of the equations involving these quantities are \[Q = mc\left( T_2 - T_1 \right), l_t = l_0 \left[ 1 + \alpha\left( T_2 - T_1 \right) \right]\] and PV = nRT.
Is the vector sum of the unit vectors \[\vec{i}\] and \[\vec{i}\] a unit vector? If no, can you multiply this sum by a scalar number to get a unit vector?
The resultant of \[\vec{A} \text { and } \vec{B}\] makes an angle α with \[\vec{A}\] and β with \[\vec{B}\],
The component of a vector is
Let \[\vec{C} = \vec{A} + \vec{B}\]
A vector \[\vec{A}\] makes an angle of 20° and \[\vec{B}\] makes an angle of 110° with the X-axis. The magnitudes of these vectors are 3 m and 4 m respectively. Find the resultant.
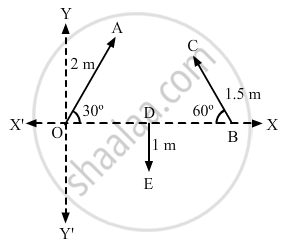
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
A spy report about a suspected car reads as follows. "The car moved 2.00 km towards east, made a perpendicular left turn, ran for 500 m, made a perpendicular right turn, ran for 4.00 km and stopped". Find the displacement of the car.
Suppose \[\vec{a}\] is a vector of magnitude 4.5 units due north. What is the vector (a) \[3 \vec{a}\], (b) \[- 4 \vec{a}\] ?
Let A1 A2 A3 A4 A5 A6 A1 be a regular hexagon. Write the x-components of the vectors represented by the six sides taken in order. Use the fact the resultant of these six vectors is zero, to prove that
cos 0 + cos π/3 + cos 2π/3 + cos 3π/3 + cos 4π/3 + cos 5π/3 = 0.
Use the known cosine values to verify the result.

Prove that \[\vec{A} . \left( \vec{A} \times \vec{B} \right) = 0\].
A curve is represented by y = sin x. If x is changed from \[\frac{\pi}{3}\text{ to }\frac{\pi}{3} + \frac{\pi}{100}\] , find approximately the change in y.
The electric current in a charging R−C circuit is given by i = i0 e−t/RC where i0, R and C are constant parameters of the circuit and t is time. Find the rate of change of current at (a) t = 0, (b) t = RC, (c) t = 10 RC.
Round the following numbers to 2 significant digits.
(a) 3472, (b) 84.16. (c)2.55 and (d) 28.5
