Advertisements
Advertisements
Question
A dimensionless quantity
Options
never has a unit,
always has a unit,
may have a unit,
does not exist.
Solution
may have a unit
Dimensionless quantities may have units.
APPEARS IN
RELATED QUESTIONS
If all the terms in an equation have same units, is it necessary that they have same dimensions? If all the terms in an equation have same dimensions, is it necessary that they have same units?
Find the dimensions of linear momentum .
Find the dimensions of
(a) angular speed ω,
(b) angular acceleration α,
(c) torque τ and
(d) moment of interia I.
Some of the equations involving these quantities are \[\omega = \frac{\theta_2 - \theta_1}{t_2 - t_1}, \alpha = \frac{\omega_2 - \omega_1}{t_2 - t_1}, \tau = F . r \text{ and }I = m r^2\].
The symbols have standard meanings.
Find the dimensions of magnetic permeability \[\mu_0\]
The relevant equation are \[F = qE, F = qvB, \text{ and }B = \frac{\mu_0 I}{2 \pi a};\]
where F is force, q is charge, v is speed, I is current, and a is distance.
Find the dimensions of Planck's constant h from the equation E = hv where E is the energy and v is the frequency.
Theory of relativity reveals that mass can be converted into energy. The energy E so obtained is proportional to certain powers of mass m and the speed c of light. Guess a relation among the quantities using the method of dimensions.
Test if the following equation is dimensionally correct:
\[V = \frac{\pi P r^4 t}{8 \eta l}\]
where v = frequency, P = pressure, η = coefficient of viscosity.
Can you have \[\vec{A} \times \vec{B} = \vec{A} \cdot \vec{B}\] with A ≠ 0 and B ≠ 0 ? What if one of the two vectors is zero?
Let \[\vec{C} = \vec{A} + \vec{B}\]
Let \[\vec{A} \text { and } \vec{B}\] be the two vectors of magnitude 10 unit each. If they are inclined to the X-axis at angle 30° and 60° respectively, find the resultant.
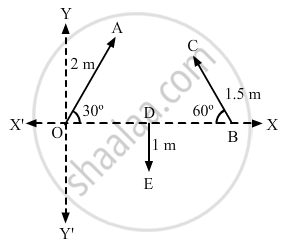
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
A mosquito net over a 7 ft × 4 ft bed is 3 ft high. The net has a hole at one corner of the bed through which a mosquito enters the net. It flies and sits at the diagonally opposite upper corner of the net. (a) Find the magnitude of the displacement of the mosquito. (b) Taking the hole as the origin, the length of the bed as the X-axis, it width as the Y axis, and vertically up as the Z-axis, write the components of the displacement vector.
Suppose \[\vec{a}\] is a vector of magnitude 4.5 units due north. What is the vector (a) \[3 \vec{a}\], (b) \[- 4 \vec{a}\] ?
Let \[\vec{a} = 2 \vec{i} + 3 \vec{j} + 4 \vec{k} \text { and } \vec{b} = 3 \vec{i} + 4 \vec{j} + 5 \vec{k}\] Find the angle between them.
Prove that \[\vec{A} . \left( \vec{A} \times \vec{B} \right) = 0\].
Give an example for which \[\vec{A} \cdot \vec{B} = \vec{C} \cdot \vec{B} \text{ but } \vec{A} \neq \vec{C}\].
Jupiter is at a distance of 824.7 million km from the Earth. Its angular diameter is measured to be 35.72˝. Calculate the diameter of Jupiter.
High speed moving particles are studied under
