Advertisements
Advertisements
प्रश्न
A carrom board (4 ft × 4 ft square) has the queen at the centre. The queen, hit by the striker moves to the from edge, rebounds and goes in the hole behind the striking line. Find the magnitude of displacement of the queen (a) from the centre to the front edge, (b) from the front edge to the hole and (c) from the centre to the hole.
उत्तर
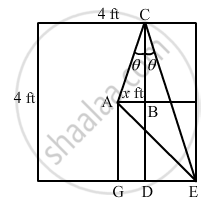
Consider that the queen is initially at point A as shown in the figure.
Let AB be x ft.
So, DE = (2 \[-\] x) ft
In ∆ABC, we have: \[\tan \theta = \frac{x}{2}\] ...(i)
Also, in ∆DCE, we have:
\[\tan \theta = \frac{\left( 2 - x \right)}{4}\] ...(ii)
From (i) and (ii), we get:
\[\frac{x}{2} = \frac{\left( 2 - x \right)}{4}\]
\[ \Rightarrow 2\left( 2 - x \right) = 4x\]
\[ \Rightarrow 4 - 2x = 4x\]
\[ \Rightarrow 6x = 4\]
\[ \Rightarrow x = \frac{2}{3}\text { ft }\]
(a) In ∆ABC, we have:
\[AC = \sqrt{{AB}^2 + {BC}^2}\]
\[= \sqrt{\left( \frac{2}{3} \right)^2 + 2^2}\]
\[ = \sqrt{\frac{4}{9} + 4} = \sqrt{\frac{40}{9}}\]
\[ = \frac{2}{3}\sqrt{10} \text { ft }\]
(b) In ∆CDE, we have:
DE \[= 2 - \frac{2}{3} = \frac{6 - 2}{3} = \frac{4}{3} \text { ft }\]
CD = 4 ft
\[\therefore CE = \sqrt{{CD}^2 + {DE}^2}\]
\[ = \sqrt{4^2 + \left( \frac{4}{3} \right)^2}\]
\[ = \frac{4}{3}\sqrt{10} \text { ft }\]
(c) In ∆AGE, we have:
\[AE = \sqrt{{AG}^2 + {GE}^2}\]
\[= \sqrt{2^2 + 2^2}\]
\[\sqrt{8} = 2\sqrt{2}\text { ft }\]
APPEARS IN
संबंधित प्रश्न
Some of the most profound statements on the nature of science have come from Albert Einstein, one of the greatest scientists of all time. What do you think did Einstein mean when he said : “The most incomprehensible thing about the world is that it is comprehensible”?
A unitless quantity
Choose the correct statements(s):
(a) All quantities may be represented dimensionally in terms of the base quantities.
(b) A base quantity cannot be represented dimensionally in terms of the rest of the base quantities.
(c) The dimensions of a base quantity in other base quantities is always zero.
(d) The dimension of a derived quantity is never zero in any base quantity.
Find the dimensions of linear momentum .
Find the dimensions of the coefficient of linear expansion α and
The height of mercury column in a barometer in a Calcutta laboratory was recorded to be 75 cm. Calculate this pressure in SI and CGS units using the following data : Specific gravity of mercury = \[13 \cdot 6\] , Density of \[\text{ water} = {10}^3 kg/ m^3 , g = 9 \cdot 8 m/ s^2\] at Calcutta. Pressure
= hpg in usual symbols.
Let I = current through a conductor, R = its resistance and V = potential difference across its ends. According to Ohm's law, product of two of these quantities equals the third. Obtain Ohm's law from dimensional analysis. Dimensional formulae for R and V are \[{\text{ML}}^2 \text{I}^{- 2} \text{T}^{- 3}\] and \[{\text{ML}}^2 \text{T}^{- 3} \text{I}^{- 1}\] respectively.
Test if the following equation is dimensionally correct:
\[h = \frac{2S cos\theta}{\text{ prg }},\]
where h = height, S = surface tension, ρ = density, I = moment of interia.
Test if the following equation is dimensionally correct:
\[V = \frac{\pi P r^4 t}{8 \eta l}\]
where v = frequency, P = pressure, η = coefficient of viscosity.
Let x and a stand for distance. Is
\[\int\frac{dx}{\sqrt{a^2 - x^2}} = \frac{1}{a} \sin^{- 1} \frac{a}{x}\] dimensionally correct?
Is a vector necessarily changed if it is rotated through an angle?
Can you add two vectors representing physical quantities having different dimensions? Can you multiply two vectors representing physical quantities having different dimensions?
A vector is not changed if
Which of the sets given below may represent the magnitudes of three vectors adding to zero?
A vector \[\vec{A}\] makes an angle of 20° and \[\vec{B}\] makes an angle of 110° with the X-axis. The magnitudes of these vectors are 3 m and 4 m respectively. Find the resultant.
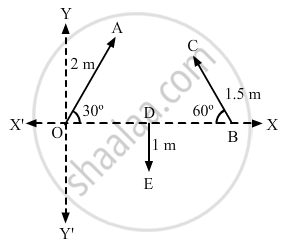
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
If \[\vec{A} = 2 \vec{i} + 3 \vec{j} + 4 \vec{k} \text { and } \vec{B} = 4 \vec{i} + 3 \vec{j} + 2 \vec{k}\] find \[\vec{A} \times \vec{B}\].
If \[\vec{A} , \vec{B} , \vec{C}\] are mutually perpendicular, show that \[\vec{C} \times \left( \vec{A} \times \vec{B} \right) = 0\] Is the converse true?
If π = 3.14, then the value of π2 is ______
High speed moving particles are studied under
