Advertisements
Advertisements
प्रश्न
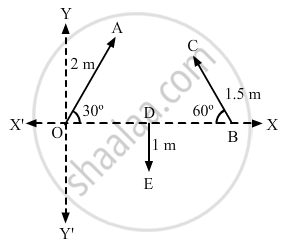
Refer to figure (2 − E1). Find (a) the magnitude, (b) x and y component and (c) the angle with the X-axis of the resultant of \[\overrightarrow{OA}, \overrightarrow{BC} \text { and } \overrightarrow{DE}\].
उत्तर
First, let us find the components of the vectors along the x and y-axes. Then we will find the resultant x and y-components.
x-component of \[\overrightarrow{OA} = 2 \cos 30^\circ= \sqrt{3}\]
x-component of \[\overrightarrow{BC}\] = 1.5 cos120°
\[= - \frac{\left( 1 . 5 \right)}{2} = - 7 . 5\]
x-component of \[\overrightarrow{DE}\] = 1 cos 270°
= 1 × 0 = 0 m
y-component of \[\overrightarrow {OA}\] = 2 sin 30° = 1
y-component of \[\overrightarrow{BC}\] = 1.5 sin 120°
\[= \frac{\left( \sqrt{3} \times 1 . 5 \right)}{2} = 1 . 3\]
y-component of \[\overrightarrow{DE}\] = 1 sin 270° = −1
x-component of resultant \[R_x = \sqrt{3} - 0 . 75 + 0 = 0 . 98 m\]
y-component of resultant Ry = 1 + 1.3 − 1 = 1.3 m
\[\therefore \text { Resultant, R }= \sqrt{\left( R_x \right)^2 + \left( R_y \right)^2}\]
\[ = \sqrt{\left( 0 . 98 \right)^2 + \left( 1 . 3 \right)^2}\]
\[ = \sqrt{0 . 96 + 1 . 69}\]
\[ = \sqrt{2 . 65}\]
\[ = 1 . 6 m\]
If it makes an angle α with the positive x-axis, then
\[\tan \alpha = \frac{\text { y -component }}{\text { x - component }}\]
\[ = \frac{1 . 3}{0 . 98} = 1 . 332\]
APPEARS IN
संबंधित प्रश्न
“Every great physical theory starts as a heresy and ends as a dogma”. Give some examples from the history of science of the validity of this incisive remark
Suppose a quantity x can be dimensionally represented in terms of M, L and T, that is, `[ x ] = M^a L^b T^c`. The quantity mass
The dimensions ML−1 T−2 may correspond to
Find the dimensions of linear momentum .
Find the dimensions of Planck's constant h from the equation E = hv where E is the energy and v is the frequency.
Find the dimensions of the coefficient of linear expansion α and
Test if the following equation is dimensionally correct:
\[h = \frac{2S cos\theta}{\text{ prg }},\]
where h = height, S = surface tension, ρ = density, I = moment of interia.
Is it possible to add two vectors of unequal magnitudes and get zero? Is it possible to add three vectors of equal magnitudes and get zero?
Can you add three unit vectors to get a unit vector? Does your answer change if two unit vectors are along the coordinate axes?
Let \[\vec{A} = 3 \vec{i} + 4 \vec{j}\]. Write a vector \[\vec{B}\] such that \[\vec{A} \neq \vec{B}\], but A = B.
Can you have \[\vec{A} \times \vec{B} = \vec{A} \cdot \vec{B}\] with A ≠ 0 and B ≠ 0 ? What if one of the two vectors is zero?
If \[\vec{A} \times \vec{B} = 0\] can you say that
(a) \[\vec{A} = \vec{B} ,\]
(b) \[\vec{A} \neq \vec{B}\] ?
The component of a vector is
Let \[\vec{C} = \vec{A} + \vec{B}\]
The magnitude of the vector product of two vectors \[\left| \vec{A} \right|\] and \[\left| \vec{B} \right|\] may be
(a) greater than AB
(b) equal to AB
(c) less than AB
(d) equal to zero.
A vector \[\vec{A}\] makes an angle of 20° and \[\vec{B}\] makes an angle of 110° with the X-axis. The magnitudes of these vectors are 3 m and 4 m respectively. Find the resultant.
A carrom board (4 ft × 4 ft square) has the queen at the centre. The queen, hit by the striker moves to the from edge, rebounds and goes in the hole behind the striking line. Find the magnitude of displacement of the queen (a) from the centre to the front edge, (b) from the front edge to the hole and (c) from the centre to the hole.
If \[\vec{A} , \vec{B} , \vec{C}\] are mutually perpendicular, show that \[\vec{C} \times \left( \vec{A} \times \vec{B} \right) = 0\] Is the converse true?
A curve is represented by y = sin x. If x is changed from \[\frac{\pi}{3}\text{ to }\frac{\pi}{3} + \frac{\pi}{100}\] , find approximately the change in y.
