Advertisements
Advertisements
प्रश्न
Two wires are kept tight between the same pair of supports. The tensions in the wires are in the ratio 2 : 1 the radii are in the ratio 3 : 1 and the densities are in the ratio 1 : 2. Find the ratio of their fundamental frequencies.
उत्तर
Given:
The tensions in the two wires are in the ratio of 2:1.
\[\Rightarrow \frac{T_1}{T_2} = 2\]
Ratio of the radii is 3:1.
\[\Rightarrow \frac{r_1}{r_2} = 3 = \frac{D_1}{D_2}\]
Density in the ratios of 1:2.
\[\Rightarrow \frac{\rho_1}{\rho_2} = \frac{1}{2}\]
Let the length of the wire be L.
\[Frequency, f = \frac{1}{LD}\sqrt{\frac{T}{\pi\rho}}\]
\[\Rightarrow f_1 = \frac{1}{L D_1}\sqrt{\frac{T_1}{\pi \rho_1}}\]
\[ \Rightarrow f_2 = \frac{1}{L D_2}\sqrt{\frac{T_2}{\pi \rho_2}}\]v
\[\therefore \frac{f_1}{f_2} = \frac{L D_2}{L D_1}\sqrt{\frac{T_1}{T_2}}\sqrt{\frac{\pi \rho_2}{\pi \rho_1}}\]
\[ \Rightarrow \frac{f_1}{f_2} = \frac{1}{3}\sqrt{\frac{2}{1} \times \frac{2}{1}}\]
\[ \Rightarrow f_1 : f_2 = 2: 3\]
APPEARS IN
संबंधित प्रश्न
A pipe 20 cm long is closed at one end. Which harmonic mode of the pipe is resonantly excited by a 430 Hz source? Will the same source be in resonance with the pipe if both ends are open? (Speed of sound in air is 340 m s–1).
Explain why (or how) In a sound wave, a displacement node is a pressure antinode and vice versa,
An open organ pipe of length L vibrates in its fundamental mode. The pressure variation is maximum
Two wires of same material are vibrating under the same tension. If the first overtone of first wire is equal to the second overtone of second wire and radius of first wire is twice the radius of the second then the ratio of length of first wire to second wire is
The number of possible natural oscillations of the air column in a pipe closed at one end of length 85 cm whose frequencies lie below 1250 Hz? (v = 340 m/s)
Water waves produced by a motor boat sailing in water are ______.
The displacement of a string is given by y (x, t) = 0.06 sin (2πx/3) cos (120 πt) where x and y are in m and t in s. The length of the string is 1.5 m and its mass is 3.0 × 10−2 kg.
- It represents a progressive wave of frequency 60 Hz.
- It represents a stationary wave of frequency 60 Hz.
- It is the result of superposition of two waves of wavelength 3 m, frequency 60 Hz each travelling with a speed of 180 m/s in opposite direction.
- Amplitude of this wave is constant.
The transverse displacement of a string (clamped at its both ends) is given by y(x, t) = 0.06 sin (2πx/3) cos (120 πt). All the points on the string between two consecutive nodes vibrate with ______.
- same frequency
- same phase
- same energy
- different amplitude.
Which of the following statements are true for a stationary wave?
- Every particle has a fixed amplitude which is different from the amplitude of its nearest particle.
- All the particles cross their mean position at the same time.
- All the particles are oscillating with same amplitude.
- There is no net transfer of energy across any plane.
- There are some particles which are always at rest.
A sonometer wire is vibrating in resonance with a tuning fork. Keeping the tension applied same, the length of the wire is doubled. Under what conditions would the tuning fork still be is resonance with the wire?
Show that when a string fixed at its two ends vibrates in 1 loop, 2 loops, 3 loops and 4 loops, the frequencies are in the ratio 1:2:3:4.
Two identical strings X and Z made of same material have tension Tx and Tz in them If their fundamental frequencies are 450 Hz and 300 Hz, respectively, then the ratio `"T"_x/"T"_"z"` is ______.
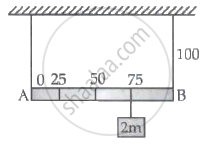
Shown in the figure is rigid and uniform one meter long rod AB held in horizontal position by two strings tied to its ends and attached to the ceiling. The rod is of mass 'm' and has another weight of mass 2m hung at a distance of 75 cm from A. The tension in the string at A is :
Two travelling waves produce a standing wave represented by the equation. y = 1.0 mm cos (1.57 cm-1) x sin (78.5 s-1)t. The node closest to the origin in the region x > 0 will be at x = ______ cm.
Two closed end pipes when sounded together produce 5 beat per second. If their length are in the ratio 100 : 101, then fundamental notes produced by them are ______.
A string 2.0 m long and fixed at its ends is driven by a 240 Hz vibrator. The string vibrates in its third harmonic mode. The speed of the wave and its fundamental frequency is ______.
