Advertisements
Advertisements
प्रश्न
When the width of the slit is made double the original width, how would this affect the size and intensity of the central diffraction band?
उत्तर
When the width of slit is made double the original width intensity will get four times of its original value.
Width of central maximum is given by,
`beta = (2Dlambda)/b`
Where, D = Distance between screen and slit,
λ = Wavelength of the light,
b = Size of slit.
So with the increase in size of slit the width of central maxima decreases. Hence, double the size of the slit would result in half the width of the central maxima.
APPEARS IN
संबंधित प्रश्न
Consider a plane wave front incident on a thin convex lens. Draw a proper diagram to show how the incident wave front traverses through the lens and after refraction focusses on the focal point of the lens, giving the shape of the emergent wave front.
Using this principle draw a diagram to show how a plane wave front incident at the interface of the two media gets refracted when it propagates from a rarer to a denser medium. Hence verify Snell's law of refraction.
Derive the law of reflection using Huygen’s Wave Theory.
Define a wavefront. Using 'Huygens' principle, draw the shape of a refracted wavefront, when a plane wave is incident on a convex lens.
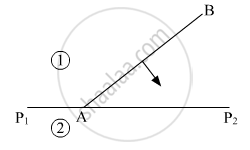
Define the term 'wavefront of light'. A plane wavefront AB propagating from a denser medium (1) into a rarer medium (2) is incident of the surface P1P2 separating the two media as shown in fig.
Using Huygen's principle, draw the secondary wavelets and obtain the refracted wavefront in the diagram.
What is the geometrical shape of the wavefront for:
- Light diverging from a point source?
- The pattern of wavefront of the light from a distant star intercepted by earth?
The inverse square law of intensity is valid for a
Wavefront means
Consider a point at the focal point of a convergent lens. Another convergent lens of short focal length is placed on the other side. What is the nature of the wavefronts emerging from the final image?
Represent diagrammatically how the incident planar wavefronts of wavelength λ pass through an aperture of size d, when d is approximately equal to λ.
