Advertisements
Advertisements
प्रश्न
Write the distance between the points A (10 cos θ, 0) and B (0, 10 sin θ).
उत्तर
We have to find the distance between A( 10 cos θ,0) and B(0 , 10 sin θ ) .
In general, the distance between A`(x_1 , y_1)` and B `(x_2 , y_2)`is given by,
`AB = sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)`
So,
`AB= sqrt((10 cos θ - 0)^2 + (0 - 10 sin θ)^2)`
` = sqrt(10^2 (sin^2 θ + cos^2 θ ) `
But according to the trigonometric identity,
`sin^2 θ + cos^2 θ = 1`
Therefore,
AB = 10
APPEARS IN
संबंधित प्रश्न
Find the distance between the following pair of points:
(a, 0) and (0, b)
Name the quadrilateral formed, if any, by the following points, and given reasons for your answers:
A(-1,-2) B(1, 0), C (-1, 2), D(-3, 0)
Find the points of trisection of the line segment joining the points:
(2, -2) and (-7, 4).
If three consecutive vertices of a parallelogram are (1, -2), (3, 6) and (5, 10), find its fourth vertex.
Find the ratio in which the pint (-3, k) divide the join of A(-5, -4) and B(-2, 3),Also, find the value of k.
In what ratio is the line segment joining the points A(-2, -3) and B(3,7) divided by the yaxis? Also, find the coordinates of the point of division.
Find the value of a, so that the point ( 3,a ) lies on the line represented by 2x - 3y =5 .
If `P(a/2,4)`is the mid-point of the line-segment joining the points A (−6, 5) and B(−2, 3), then the value of a is
Show that A(-4, -7), B(-1, 2), C(8, 5) and D(5, -4) are the vertices of a
rhombus ABCD.
Write the coordinates of the point dividing line segment joining points (2, 3) and (3, 4) internally in the ratio 1 : 5.
Find the values of x for which the distance between the point P(2, −3), and Q (x, 5) is 10.
If A (1, 2) B (4, 3) and C (6, 6) are the three vertices of a parallelogram ABCD, find the coordinates of fourth vertex D.
If Points (1, 2) (−5, 6) and (a, −2) are collinear, then a =
If A(4, 9), B(2, 3) and C(6, 5) are the vertices of ∆ABC, then the length of median through C is
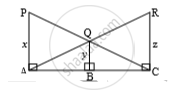
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
Points (1, – 1), (2, – 2), (4, – 5), (– 3, – 4) ______.
If the perpendicular distance of a point P from the x-axis is 5 units and the foot of the perpendicular lies on the negative direction of x-axis, then the point P has ______.
Abscissa of a point is positive in ______.
Find the coordinates of the point whose abscissa is 5 and which lies on x-axis.
The distance of the point (–4, 3) from y-axis is ______.
