Advertisements
Advertisements
Question
A cubical block of ice floating in water has to support a metal piece weighing 0.5 kg. Water can be the minimum edge of the block so that it does not sink in water? Specific gravity of ice = 0.9.
Solution
Given:
Specific gravity of ice, ρice = 0.9 gm/cc
Weight of the metal piece, m = 500 g
Density of water, \[\rho_w\] = 103 kg/m3
Let x be the minimum edge of the ice block in cm.
We have:
mg + Wice = U
Here,
U = Upward thrust
Wice = Weight of the ice
\[\text{Thus, we have: }\]
\[0 . 5 \times \text{ g + x}^3 \times \rho_{\text{ ice }} \times \text{ g = x} ^3 \times \rho_w \times g \left[ \text{ Volume of the liquid displaced = x}^3 \right]\]
\[ \Rightarrow 0 . 5 \times {10}^3 + x^3 \times (0 . 9) = x^3 \times 1\]
\[ \Rightarrow x^3 \times (0 . 1) = (0 . 5) \times {10}^3 \]
\[ \Rightarrow x^3 = 5 \times {10}^3 \]
\[ \Rightarrow x = 17 . 09 \text{ cm}\]
\[ \Rightarrow x = 17 \text{ cm }\]
APPEARS IN
RELATED QUESTIONS
Explain why Water on a clean glass surface tends to spread out while mercury on the same surface tends to form drops. (Put differently, water wets glass while mercury does not.)
Show that the surface tension of a liquid is numerically equal to the surface energy per unit
area.
State any two characteristics of the angle of contact
The excess pressure inside a soap bubble is twice the excess pressure inside a second soap bubble. The volume of the first bubble is n times the volume of the second where n is
A 20 cm long capillary tube is dipped in water. The water rises up to 8 cm. If the entire arrangement is put in a freely falling elevator, the length of water column in the capillary tube will be
The rise of a liquid in a capillary tube depends on
(a) the material
(b) the length
(c) the outer radius
(d) the inner radius of the tube
Consider a small surface area of 1 mm2 at the top of a mercury drop of radius 4.0 mm. Find the force exerted on this area (a) by the air above it (b) by the mercury below it and (c) by the mercury surface in contact with it. Atmospheric pressure = 1.0 × 105 Pa and surface tension of mercury = 0.465 N m−1. Neglect the effect of gravity. Assume all numbers to be exact.
A barometer is constructed with its tube having radius 1.0 mm. Assume that the surface of mercury in the tube is spherical in shape. If the atmospheric pressure is equal to 76 cm of mercury, what will be the height raised in the barometer tube? The contact angle of mercury with glass = 135° and surface tension of mercury = 0.465 N m−1. Density of mercury = 13600 kg m−3.
The energy stored in a soap bubble of diameter 6 cm and T = 0.04 N/m is nearly ______.
A u-tube is made up of capillaries of bore 1 mm and 2 mm respectively. The tube is held vertically and partially filled with a liquid of surface tension 49 dyne/cm and zero angles of contact. Calculate the density of the liquid, if the difference in the levels of the meniscus is 1.25 cm. take g = 980 cm/s2
The wettability of a surface by a liquid depends primarily on
A capillary of diameter d mm is dipped in water such that the water rises to a height of 30 mm. If the radius of the capillary is made `(2/3)` of its previous value, then compute the height up to which water will rise in the new capillary?
Water rises in a capillary tube of radius r upto a height h. The mass of water in a capillary is m. The mass of water that will rise in a capillary of radius `"r"/4` will be ______.
The surface tension of the two liquids is respectively 20 and 60 dyne cm-1. The liquids drop from the ends of two tubes of the same radius. The ratio of the weights of the two drops is ______
The excess of pressure, due to surface tension, on a spherical liquid drop of radius 'R' is proportional to ______.
The upward force of 105 dyne due to surface tension is balanced by the force due to the weight of the water column and 'h' is the height of water in the capillary. The inner circumference of the capillary is ______.
(surface tension of water = 7 × 10-2 N/m)
The wear and tear in the machine part is due to ______.
Is surface tension a vector?
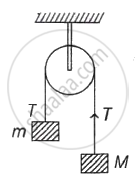
Two blocks of masses m and M are connected by means of a metal wire of cross-sectional area A passing over a frictionless fixed pully as shown in the figure. The system is then released. If M = 2m, then the stress produced in the wire is ______.
The surface tension of soap solution is 25 × 10-3 Nm-1. The excess of pressure inside a soap bubble of diameter 1 cm is ______.
