Advertisements
Advertisements
Question
A line PQ is drawn parallel to the side BC of ΔABC which cuts side AB at P and side AC at Q. If AB = 9.0 cm, CA = 6.0 cm and AQ = 4.2 cm, find the length of AP.
Solution
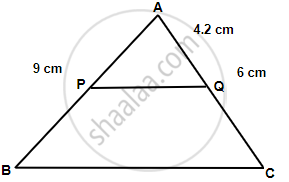
In ΔAPQ and ΔABC,
∠APQ = ∠ABC ...(Since PQ || BC, so the angles are corresponding angles)
∠PAQ = ∠BAC ...(Common angle)
ΔAPQ ∼ ΔABC ...(AA criterion for similarity)
`=> (AP)/(AB) = (AQ)/(AC)`
`=> (AP)/9 = 4.2/6`
`=> AP = (4.2 xx 9)/6`
`=>` AP = 6.3 cm
APPEARS IN
RELATED QUESTIONS
In figure, if ∠A = ∠C, then prove that ∆AOB ~ ∆COD

In the given figure, ∠PQR = ∠PST = 90° ,PQ = 5cm and PS = 2cm.
(i) Prove that ΔPQR ~ ΔPST.
(ii) Find Area of ΔPQR : Area of quadrilateral SRQT.

In MBC, DE is drawn parallel to BC. If AD: DB=2:3, DE =6cm and AE =3.6cm, find BC and AC.
ΔABC is enlarged, with a scale factor 5. Find: A'B', if AB = 4cm
A plot of land of area 20km2 is represented on the map with a scale factor of 1:200000. Find: The ground area in km2 that is represented by 2cm2 on the map.
If in triangles PQR and XYZ, `"PQ"/"XY" = "QR"/"ZX"` then they will be similar if
ΔPQR ~ ΔSUV. Write pairs of congruent angles
In ΔABC, AP ⊥ BC and BQ ⊥ AC, B−P−C, A−Q−C, then show that ΔCPA ~ ΔCQB. If AP = 7, BQ = 8, BC = 12, then AC = ?

In ΔCPA and ΔCQB
∠CPA ≅ [∠ ______] ...[each 90°]
∠ACP ≅ [∠ ______] ...[common angle]
ΔCPA ~ ΔCQB ......[______ similarity test]
`"AP"/"BQ" = (["______"])/"BC"` .......[corresponding sides of similar triangles]
`7/8 = (["______"])/12`
AC × [______] = 7 × 12
AC = 10.5
In the given figure ΔABC ~ ΔPQR. The value of x is
 |
 |

In ΔABC, DE || BC (as shown in the figure), If AD = 4 cm, AB = 9 cm and AC = 13.5 cm, then the length of EC is ______.
