Advertisements
Advertisements
Question
In the given figure, PQ || AB; CQ = 4.8 cm QB = 3.6 cm and AB = 6.3 cm. Find : If AP = x, then the value of AC in terms of x.

Solution
In ΔCPQ and ΔCAB,
∠PCQ = ∠ACB ...(Since PQ || AB, so the angles are corresponding angles)
∠C = ∠C ...(Common angle)
∴ ΔCPQ ∼ ΔCAB ...(AA criterion for similarity)
`=> (CP)/(AC) = (CQ)/(CB)`
`=> (CP)/(AC) = (4.8)/(8.4) = 4/7 `
So, if AC is 7 parts, and CP is 4 parts, then PA is 3 parts.
Given, AP = x
or 3 parts = x
`=>` 1 part = `x/3`
`=>` 7 parts = `(7x)/3`
Hence, AC = `(7x)/3`
APPEARS IN
RELATED QUESTIONS
In the given figure ABC is a triangle with ∠EDB = ∠ACB. Prove that Δ ABC ~ Δ EBD. If BE = 6 cm, EC = 4 cm, BD = 5 cm. And area of Δ BED = 9 cm2. Calculate the
(1) length of AB
(2) area of Δ ABC

State, true or false:
Two similar polygons are necessarily congruent.
On a map drawn to a scale of 1 : 2,50,000; a triangular plot of land has the following measurements : AB = 3 cm, BC = 4 cm and ∠ABC = 90°.
Calculate : the actual lengths of AB and BC in km.
In the following figure, point D divides AB in the ratio 3 : 5. Find :
- `(AE)/(EC)`
- `(AD)/(AB)`
- `(AE)/(AC)`
Also, if: - DE = 2.4 cm, find the length of BC.
- BC = 4.8 cm, find the length of DE.

A line PQ is drawn parallel to the side BC of ΔABC which cuts side AB at P and side AC at Q. If AB = 9.0 cm, CA = 6.0 cm and AQ = 4.2 cm, find the length of AP.
In the figure, DE || AC and DC || AP. Prove that `"BE"/"EC" = "BC"/"CP"`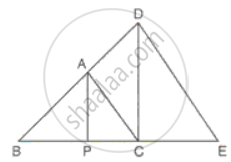
D and E are points on the sides AB and AC of ΔABC such that DE | | BC and divides ΔABC into two parts, equal in area. Find `"BD"/"AB"`.
Find the scale factor in each of the following and state the type of size transformation:
Image length = 8cm, Actual length = 20cm.
A map is drawn to scale of 1:20000. Find: The distance on the map representing 4km
A flag pole 15 m high casts a shadow of 3 m at 10 a.m. The shadow cast by a building at the same time is 18.6 m. The height of the building is
