Advertisements
Advertisements
Question
A mild steel wire of length 1.0 m and cross-sectional area 0.50 × 10–2 cm2 is stretched, well within its elastic limit, horizontally between two pillars. A mass of 100 g is suspended from the mid-point of the wire. Calculate the depression at the midpoint.
Solution 1
Let AB be a mild steel wire of length 2L = lm and its cross-section area A = 0.50 x 10-2 cm2. A mass m = 100 g = 0.1 kg is suspended at mid-point C of wire as shown in figure. Let x be the depression at mid-point i.e., CD = x
`:. AD = DB = sqrt((AC^2 + CD^2)) = sqrt(L^2+x^2)`
:. Increase in length `triangleL = (AD+DB) - AB = 2sqrt(L^2+x^2) - 2L`
`= 2L[(1+x^2/L^2)^(1/2) - 1] = 2L.x^2/(2L^2) = x^2/L`
:. Longitudinal strain = `(triangleL)/(2L) = x^2/(2L^2)`
If T be the tension in the wire as shown figure then in equilibrium `2T cos theta = mg`
or `T = "mg"/(2costheta)`
`= "mg"/(2 x/(sqrt(x^2+L^2))) = (mgsqrt(x^2+L^2))/(2x) = "mgL"/(2x)`
:. Stress = `T/A = "mgL"/(2 x A)`

As Young's modulus Y = `"stress"/"strain"`
`=((mgL)/(2 x A))/((x^2)/(2L^2)) = (mgL)/(2 x A) xx (2L^2)/x^2 = (mgL^3)/(Ax^3)`
`=> x = [(mgL^3)/(YA)]^(1/3) = L[(mg)/(YA)]^(1/3)`
`= 1/2[(0.1xx9.8)/(2xx10^11xx0.50xx10^(-2)xx10^(-4))]^(1/3) = 1.074 xx 10^(-2) m`
= 1.074 cm ≈ 1.07 cm or 0.01 m
Solution 2

Length of the steel wire = 1.0 m
Area of cross-section, A = 0.50 × 10–2 cm2 = 0.50 × 10–6 m2
A mass 100 g is suspended from its midpoint.
m = 100 g = 0.1 kg
Hence, the wire dips, as shown in the given figure.
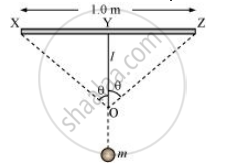
Original length = XZ
Depression = l
The length after mass m is attached to the wire = XO + OZ
Increase in the length of the wire:
Δl = (XO + OZ) – XZ
Where,
XO = OZ = `[(0.5)^2 + l^2]^(1/2)`
`:.triangle l = 2[(0.5)^2 + (l)^2]^(1/2) - 1.0`
`= 2 xx 0.5 [1+(l/0.5)^2]^(1/2) - 1.0`
Expanding and neglecting higher terms, we get
`triangle l = l^2/0.5`
`"Strain" = "Increase in length"/"Original length"`
Let T be the tension in the wire.
∴mg = 2T cosθ
Using the figure, it can be written as:
`cos theta = l/((0.5)^2 + l^2)^(1/2)`
`= l/ ((0.5)(1+(l/0.5)^2)^(1/2))`
Expanding the expression and eliminating the higher terms:
`cos theta = l/((0.5)(1+l^2/(2(0.5)^2)))`
`(1+l^2/(0.5))=~1` for small l
`:. cos theta = l/(0.5)`
`:. T = (mg)/(2(l/(0.5))) = (mgxx 0.5)/(2l) = (mg)/(4l)`
`"Stress" = "Tension"/"Area" = (mg)/(4lxxA)`
`Y = (mg xx 0.5)/(4lxxAxxl^2)`
`l = sqrt((mgxx0.5)/(4YA)) `
Young’s modulus of steel, Y = 2 x 1011 Pa
`:.l = sqrt((0.1xx9.8xx0.5)/(4xx2xx10^11xx0.50xx10^(-6)))`
= 0.0106 m
Hence, the depression at the midpoint is 0.0106 m.
APPEARS IN
RELATED QUESTIONS
A rigid bar of mass 15 kg is supported symmetrically by three wires each 2.0 m long. Those at each end are of copper and the middle one is of iron. Determine the ratio of their diameters if each is to have the same tension.
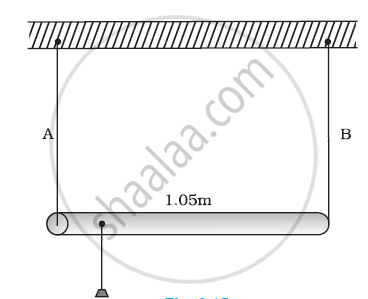
A rod of length 1.05 m having negligible mass is supported at its ends by two wires of steel (wire A) and aluminium (wire B) of equal lengths as shown in Figure. The cross-sectional areas of wires A and B are 1.0 mm2 and 2.0 mm2, respectively. At what point along the rod should a mass m be suspended in order to produce (a) equal stresses and (b) equal strains in both steel and aluminium wires.
The ratio stress/strain remain constant for small deformation of a metal wire. When the deformation is made larger, will this ratio increase or decrease?
A steel blade placed gently on the surface of water floats on it. If the same blade is kept well inside the water, it sinks. Explain.
Answer in one sentence.
How should be a force applied on a body to produce shearing stress?
Modulus of rigidity of ideal liquids is ______.
A rectangular frame is to be suspended symmetrically by two strings of equal length on two supports (Figure). It can be done in one of the following three ways;
| (a) |  |
| (b) |  |
| (c) |  |
The tension in the strings will be ______.
A rod of length l and negligible mass is suspended at its two ends by two wires of steel (wire A) and aluminium (wire B) of equal lengths (Figure). The cross-sectional areas of wires A and B are 1.0 mm2 and 2.0 mm2, respectively.
(YAl = 70 × 109 Nm−2 and Ysteel = 200 × 109 Nm–2)
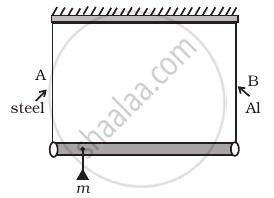
- Mass m should be suspended close to wire A to have equal stresses in both the wires.
- Mass m should be suspended close to B to have equal stresses in both the wires.
- Mass m should be suspended at the middle of the wires to have equal stresses in both the wires.
- Mass m should be suspended close to wire A to have equal strain in both wires.
The area of the cross-section of the rope used to lift a load by a crane is 2.5 × 10-4m2. The maximum lifting capacity of the crane is 10 metric tons. To increase the lifting capacity of the crane to 25 metric tons, the required area of cross-section of the rope should be ______.
(take g = 10 ms-2)
Answer in one sentence:
What is an elastomer?
