Advertisements
Advertisements
Question
A particle moves from a point \[\overrightarrow{r}_1 = \left( 2 m \right) \overrightarrow{ i } + \left( 3 m \right) \overrightarrow{ j } \] to another point
\[\overrightarrow{r}_2 = \left( 3 m \right) \overrightarrow{ i } + \left( 2 m \right) \overrightarrow{ j } \] acts on it. Find the work done by the force on the particle during the displacement.
Solution
Initial position vector, \[\vec{r_1} = 2 \vec{i} + 3 \vec{j}\] Final position vector, \[\vec{r}_2 = 3 \vec{i} + 2 \vec{j}\]
So, displacement vector,
\[\vec{r} = \vec{r}_2 - \vec{r}_1 \]
\[ = \left( 3 \vec{i} + 2 \vec{j} \right) - \left( 2 \vec{i} + \vec{j} \right)\]
\[ = \vec{i} - \vec{j} \]
\[\text{ Force acting on the particle } , \vec{F} = 5 \vec{i} + 5 \vec{j} \]
\[\text{ So, work done } = \vec{F} \cdot \vec{S} = 5 \times 1 + 5 \left( - 1 \right) = 0\]
APPEARS IN
RELATED QUESTIONS
A lawyer alleges in court that the police had forced his client to issue a statement of confession. What kind of force is this ?
List all the forces acting on the block B in figure.
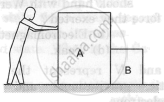
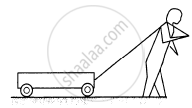
Figure shows a boy pulling a wagon on a road. List as many forces as you can which are relevant with this figure. Find the pairs of forces connected by Newton's third law of motion.
A 60 kg man pushes a 40 kg man by a force of 60 N. The 40 kg man has pushed the other man with a force of
A neutron exerts a force on a proton which is
(a) gravitational
(b) electromagnetic
(c) nuclear
(d) weak
If all matters were made of electrically neutral particles such as neutrons,
(a) there would be no force of friction
(b) there would be no tension in the string
(c) it would not be possible to sit on a chair
(d) the earth could not move around the sun.
Calculate the force with which you attract the earth.
A satellite is projected vertically upwards from an earth station. At what height above the earth's surface will the force on the satellite due to the earth be reduced to half its value at the earth station? (Radius of the earth is 6400 km.)
The magnetic force on a charged particle is always perpendicular to its velocity. Can the magnetic force change the velocity of the particles? Speed of the particle?
The work done by all the forces (external and internal) on a system equals the change in ______.
A constant force of 2⋅5 N accelerates a stationary particle of mass 15 g through a displacement of 2⋅5 m. Find the work done and the average power delivered.
A particle of mass m moves on a straight line with its velocity varying with the distance travelled, according to the equation \[\nu = a\sqrt{x}\] , where a is a constant. Find the total work done by all the forces during a displacement from \[x = 0 \text{ to } x - d\] .
A block of mass 2 kg kept at rest on an inclined plane of inclination 37° is pulled up the plane by applying a constant force of 20 N parallel to the incline. The force acts for one second. Show that the work done by the applied force does not exceed 40 J.
A block of mass 2 kg kept at rest on an inclined plane of inclination 37° is pulled up the plane by applying a constant force of 20 N parallel to the incline. The force acts for one second. Find the kinetic energy of the block at the instant the force ceases to act. Take g = 10 m/s2.
The 200 m free-style women's swimming gold medal at Seoul Olympics in 1988 was won by Heike Friendrich of East Germany when she set a new Olympic record of 1 minute and 57⋅56 seconds. Assume that she covered most of the distance with a uniform speed and had to exert 460 W to maintain her speed. Calculate the average force of resistance offered by the water during the swim.
A uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is μ . Find the work done by friction during the period the chain slips off the table.
The work done by an applied variable force, F = x + x3 from x = 0 m to x = 2m, where x is displacement, is:
A body of mass 0.5 kg travels in a straight line with velocity v = a x3/2 where a = 5 m–1/2s–1. The work done by the net force during its displacement from x = 0 to x = 2 m is ______.
A body is moving unidirectionally under the influence of a source of constant power supplying energy. Which of the diagrams shown in figure correctly shows the displacement-time curve for its motion?
A block of mass m is taken from A to B slowly under the action of a constant force R Work done by this force is ______.

