Advertisements
Advertisements
Question
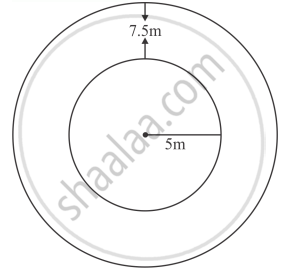
A well with 10 m inside diameter is dug 8.4 m deep. Earth taken out of it is spread all around it to a width of 7.5 m to form an embankment. Find the height of the embankment.
Solution
Radius of well
`r = 10/2`
` = 5m`
Depth of well h = 8.4 m
Clearly,
Volume of earth dugout
`= pi(5)^2 xx 8.4`
`= pi xx 25 xx 8.4`
` =(22 xx 25 xx 8.4)/7 m^3`
Let h' be the height of embankment
Clearly,
Embankment forms a cylindrical shell whose inner and outer radius are 5 m and 12.5 m respectively.
∴ Volume of the embankment
`= pi{(12.5)^2 - (5)^2} xx h``
` = pi xx 17.5 xx 7.5 xx h' m^3`
But, volume of earth dugout = volume of the embankment
`(22 xx 25 xx 8.4)/7 = 22/7 xx 17.5 xx 7.5 xx h`
` h = (25 xx 8.4)/(17.5 xx 7.5)`
`h` = 1.6 m`
APPEARS IN
RELATED QUESTIONS
A bucket is in the form of a frustum of a cone with a capacity of 12308.8 cm3 of water.The radii of the top and bottom circular ends are 20 cm and 12 cm respectively. Find the height of the bucket and the area of the metal sheet used in its making. (Use 𝜋 = 3.14).
A rectangular sheet of paper 40 cm × 22 cm, is rolled to form a hollow cylinder of height 40 cm. The radius of the cylinder (in cm) is ______.
A wooden toy was made by scooping out a hemisphere of same radius from each end of a solid cylinder. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the volume of wood in the toy. `[\text{Use}pi 22/7]`
Choose the correct answer of the following question:
The radii of the circular ends of a bucket of height 40 cm are 24 cm and 15 cm. The slant height (in cm) of the bucket is
The ratio of the total surface area to the lateral surface area of a cylinder with base radius 80 cm and height 20 cm is
If the radii of the ends of a bucket are 5 cm and 15 cm and it is 24 cm high, then its surface area is
Assertion (A)
The curved surface area of a cone of base radius 3 cm and height 4 cm is 15π cm2.\
Reason (R)
Volume of a cone = πr2h
- Both Assertion (A) and Reason (R) are true and Reason (R) is a correct explanation of Assertion (A).
- Both Assertion (A) and Reason (R) are true but Reason (R) is not a correct explanation of Assertion (A).
- Assertion (A) is true and Reason (R) is false.
- Assertion (A) is false and Reason (R) is true.
How many solid cylinders of radius 6 cm and height 12 cm can be made by melting a solid sphere of radius 18 cm?
Activity: Radius of the sphere, r = 18 cm
For cylinder, radius R = 6 cm, height H = 12 cm
∴ Number of cylinders can be made =`"Volume of the sphere"/square`
`= (4/3 pir^3)/square`
`= (4/3 xx 18 xx 18 xx 18)/square`
= `square`
______ surface area of room = area of 4 walls.
A rectangular examination hall having seats for 500 candidates has to be built so as to allow 4 cubic metres of air and 0.5 square metres of floor area per candidate. If the length of hall be 25 m, find the height and breadth of the hall.
