Advertisements
Advertisements
Question
ABC is an isosceles triangle right angled at C. Prove that AB2 = 2AC2
Solution
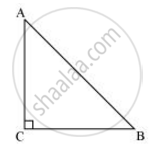
Given that ΔABC is an isosceles triangle.
∴ AC = CB
Applying Pythagoras theorem in ΔABC (i.e., right-angled at point C), we obtain
AC2 + CB2 = AB2
=> AC2+ AC2 = AB2 (AC = CB)
⇒ 2AC2 = AB2
APPEARS IN
RELATED QUESTIONS
An aeroplane leaves an airport and flies due north at a speed of 1,000 km per hour. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1,200 km per hour. How far apart will be the two planes after `1 1/2` hours?
In an equilateral triangle, prove that three times the square of one side is equal to four times the square of one of its altitudes.
Identify, with reason, if the following is a Pythagorean triplet.
(11, 60, 61)
Two poles of heights 6 m and 11 m stand vertically on a plane ground. If the distance between their feet is 12 m;
find the distance between their tips.
Find the Pythagorean triplet from among the following set of numbers.
3, 4, 5
The sides of the triangle are given below. Find out which one is the right-angled triangle?
11, 60, 61
A ladder 25m long reaches a window of a building 20m above the ground. Determine the distance of the foot of the ladder from the building.
If ‘l‘ and ‘m’ are the legs and ‘n’ is the hypotenuse of a right angled triangle then, l2 = ________
In the figure, find AR
If ΔABC ~ ΔPQR, `("ar" triangle "ABC")/("ar" triangle "PQR") = 9/4` and AB = 18 cm, then the length of PQ is ______.
