Advertisements
Advertisements
Question
ABCD is a parallelogram. P and Q are mid-points of AB and CD. Prove that APCQ is also a parallelogram.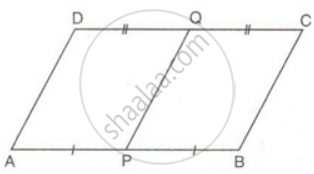
Solution
In quandrilateral APCQ,
AP || QC ...(since AB || CD)
AP = `(1)/(2)"AB"` ...(given)
CQ = `(1)/(2)"CD"` ...(given)
But AB = CD
⇒ AP = CQ
Therefore, APCQ is a parallelogram.
APPEARS IN
RELATED QUESTIONS
ABCD is a parallelogram. P and T are points on AB and DC respectively and AP = CT. Prove that PT and BD bisect each other.
PQRS is a parallelogram. PQ is produced to T so that PQ = QT. Prove that PQ = QT. Prove that ST bisects QR.
Prove that if the diagonals of a parallelogram are equal then it is a rectangle.
Prove that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides, and is equal to half the difference of these sides.
ABCD is a trapezium in which side AB is parallel to side DC. P is the mid-point of side AD. IF Q is a point on the Side BC such that the segment PQ is parallel to DC, prove that PQ = `(1)/(2)("AB" + "DC")`.
In the given figure, PQRS is a parallelogram in which PA = AB = Prove that: SAQB is a parallelogram.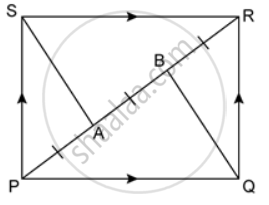
In the given figure, PQRS is a trapezium in which PQ ‖ SR and PS = QR. Prove that: ∠PSR = ∠QRS and ∠SPQ = ∠RQP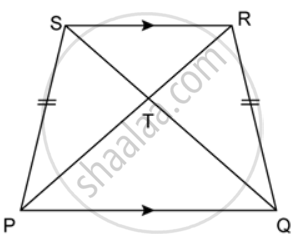
Prove that the diagonals of a square are equal and perpendicular to each other.
Prove that the diagonals of a parallelogram divide it into four triangles of equal area.
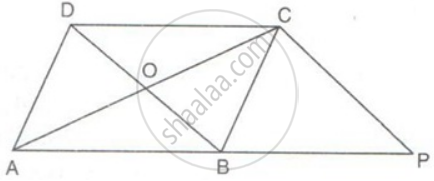
In the figure, ABCD is a parallelogram and CP is parallel to DB. Prove that: Area of OBPC = `(3)/(4)"area of ABCD"`