Advertisements
Advertisements
Question
ABCD is a rectangle formed by the points A(-1, -1), B(-1, 4), C(5, 4) and D(5, -1). P, Q, R and S are the midpoints of AB, BC, CD and DA respectively. Is the quadrilateral PQRS a square? a rectangle? or a rhombus? Justify your answer.
Solution

P is the mid point of side AB
THerefore the coordinates of P are ((-1-1)/2,(-1+4)/2) = (-1, 3/2)
Similary the coordinates of Q , R and S are (2,4),(5, 3/2), and (2, -1) respectilvely
Length of PQ =`sqrt((-1-2)^2 + (3/2-4)^2) =sqrt(9+25/4)= sqrt(61/4)`
Length of QR =`sqrt((2-5)^2 + (4-3/2)^2) =sqrt(9+25/4)= sqrt(61/4)`
Length of RS =`sqrt((5-2)^2 + (3/2+1)^2) =sqrt(9+25/4)= sqrt(61/4)`
Length of SP =`sqrt((2+1)^2 + (-1-3/2)^2) =sqrt(9+25/4)= sqrt(61/4)`
Length of PR =`sqrt((-1-5)^2 + (3/2-3/2)^2) = 6`
Length of QS = `sqrt((2-2)^2 + (4+1)^2) = 5`
It can be observed that all sides of the given quadrilateral are of the same measure. However, the diagonals are of different lengths. Therefore, PQRS is a rhombus.
APPEARS IN
RELATED QUESTIONS
If the points A(−1, −4), B(b, c) and C(5, −1) are collinear and 2b + c = 4, find the values of b and c.
In each of the following find the value of 'k', for which the points are collinear.
(7, -2), (5, 1), (3, -k)
Find values of k if area of triangle is 4 square units and vertices are (k, 0), (4, 0), (0, 2)
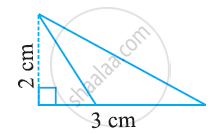
Find the area of the following triangle:
Four points A (6, 3), B (−3, 5), C(4, −2) and D (x, 3x) are given in such a way that `(ΔDBG) /(ΔABG)=1/2,` find x
Prove that the points (a, b), (a1, b1) and (a −a1, b −b1) are collinear if ab1 = a1b.
In ☐ABCD, l(AB) = 13 cm, l(DC) = 9 cm, l(AD) = 8 cm, find the area of ☐ABCD.

The table given below contains some measures of the right angled triangle. Find the unknown values.
| Base | Height | Area |
| ? | 12 m | 24 sq.m |
The base and the corresponding altitude of a parallelogram are 10 cm and 3.5 cm, respectively. The area of the parallelogram is 30 cm2.
Let a vector `αhati + βhatj` be obtained by rotating the vector `sqrt(3)hati + hatj` by an angle 45° about the origin in counter-clockwise direction in the first quadrant. Then the area of triangle having vertices (α, β), (0, β) and (0, 0) is equal to ______.
