Advertisements
Advertisements
Question
An electron and a proton are moving under the influence of mutual forces. In calculating the change in the kinetic energy of the system during motion, one ignores the magnetic force of one on another. This is because ______.
Options
the two magnetic forces are equal and opposite, so they produce no net effect.
the magnetic forces do no work on each particle.
the magnetic forces do equal and opposite (but non-zero) work on each particle.
the magenetic forces are necessarily negligible.
Solution
An electron and a proton are moving under the influence of mutual forces. In calculating the change in the kinetic energy of the system during motion, one ignores the magnetic force of one on another. This is because the magnetic forces do no work on each particle.
Explanation:
The work-energy theorem states that net work done equals final kinetic energy - the initial kinetic energy of the item.
The following equation demonstrates the relationship between work and kinetic energy:
∑W = K2 – K1v
As the electron and proton move under the influence of mutual interactions, the magnetic forces will be perpendicular to their motion, acting as a centripetal force for the particle.
As a result of performing the uniform circular motion in this manner, the particle's speed remains constant.
As a result, the particle's kinetic energy remains unchanged.
As a result, these forces perform no work.
`vecF_m = q(vecv xx vecB) * F_m` (magnetic force) will be perpendicular to both B and v, where B represents the external magnetic field and v represents particle velocity.
That is why the magnetic pull of one particle on another is ignored.
APPEARS IN
RELATED QUESTIONS
The US athlete Florence Griffith-Joyner won the 100 m sprint gold medal at Seoul Olympics in 1988, setting a new Olympic record of 10⋅54 s. Assume that she achieved her maximum speed in a very short time and then ran the race with that speed till she crossed the line. Take her mass to be 50 kg. Calculate the kinetic energy of Griffith-Joyner at her full speed.
A scooter company gives the following specifications about its product:
Weight of the scooter − 95 kg
Maximum speed − 60 km/h
Maximum engine power − 3⋅5 hp
Pick up time to get the maximum speed − 5 s
Check the validity of these specifications.
A simple pendulum consists of a 50 cm long string connected to a 100 g ball. The ball is pulled aside so that the string makes an angle of 37° with the vertical and is then released. Find the tension in the string when the bob is at its lowest position.
Following figure following shows a smooth track, a part of which is a circle of radius R. A block of mass m is pushed against a spring of spring constant k fixed at the left end and is then released. Find the initial compression of the spring so that the block presses the track with a force mg when it reaches the point P, where the radius of the track is horizontal.

Figure ( following ) shows a smooth track which consists of a straight inclined part of length l joining smoothly with the circular part. A particle of mass m is projected up the incline from its bottom. Find the minimum projection-speed \[\nu_0\] for which the particle reaches the top of the track.
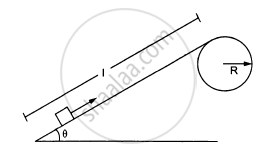
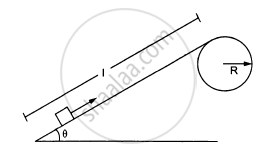
Figure ( following ) shows a smooth track which consists of a straight inclined part of length l joining smoothly with the circular part. A particle of mass m is projected up the incline from its bottom.Assuming that the projection-speed is only slightly greater than \[\nu_0\] , where will the block lose contact with the track?
A chain of length l and mass m lies on the surface of a smooth sphere of radius R > l with one end tied to the top of the sphere. Find the gravitational potential energy of the chain with reference level at the centre of the sphere.
A chain of length l and mass m lies on the surface of a smooth sphere of radius R > l with one end tied to the top of the sphere. Find the tangential acceleration \[\frac{d\nu}{dt}\] of the chain when the chain starts sliding down.
Give example of a situation in which an applied force does not result in a change in kinetic energy.
A particle moves in one dimension from rest under the influence of a force that varies with the distance travelled by the particle as shown in the figure. The kinetic energy of the particle after it has travelled 3 m is ______.

