Advertisements
Advertisements
Question
An electron in an atom is revolving around the nucleus in a circular orbit of a radius of 5.3 x 10-11 m, with a speed of 2 x 106 m/s. Find the resultant orbital magnetic moment and angular momentum of the electron. [e = 1.6 x 10-19 C, me= 9.1 x 10-31 kg]
Solution 1
- Data: r = 5.3 × 10-11 m, v = 2 × 106 m/s,
e = 1.6 × 10-19 C, me = 9.1 × 10-31 kg
The orbital magnetic moment of the electron is
`"M"_0 = 1/2`evr
`= 1/2(1.6 xx 10^-19)(2xx10^6)(5.3 xx 10^-11)`
= 8.48 × 10-24 A.m2
The angular momentum of the electron is
L0 = mevr
= (9.1 × 10-31)(2 × 106)(5.3 × 10-11)
= 96.46 × 10-36 = 9.646 × 10-35 kg.m2/s
Solution 2
Given:
r = `5.3 xx 10^-11` m,
v = `2 xx 10^6` ms-1,
e = `1.6 xx 10^-19` C,
me = `9.1 xx 10^-31` kg
To find:
- Orbital magnetic moment (morb)
- Angular momentum of electron
Formulae:
- morb = `"evr"/2`
- L = mvr
Calculation:
From formula (i),
morb = `(1.6 xx 10^-19 xx 2 xx 10^6 xx 5.3 xx 10^-11)/2`
= `1.6 xx 5.3 xx 10^-24`
= `8.48 xx 10^-24` Am2
From formula (ii),
L = `9.1 xx 10^-31 xx 2 xx 10^6 xx 5.3 xx 10^-11`
= `96.46 xx 10^-36`
∴ L ≈ `underline(9.646 xx 10^-35)` kgm2/s
- Orbital magnetic moment is `8.48 xx 10^-24` Am2.
- Angular momentum of electron is `9.646 xx 10^-35` kgm2/s.
APPEARS IN
RELATED QUESTIONS
What is stated in terms of Bohr magneton?
Explain the origin of magnetism in material, hence find a magnetic moment of an electron revolving around the nucleus of an atom.
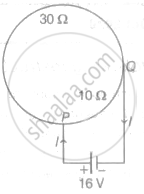
A circular coil of radius R has a resistance of 40 Ω. Figure shows two points P and Q on the circumference separated by a distance `(pi"R")/2`, which are connected to a 16 V battery with internal resistance of 0.5 Ω. What is the value of current I flowing through the circuit?
The magnetic moment of electrons due to orbital motion is proportional to ______.
(n = principal quantum numbers)
The S.I. unit of gyromagnetic ratio is ______
The Gyromagnetic ratio of the electron revolving in a circular orbit of a hydrogen atom is 8.8 x 1010 C kg-1. What is the mass of the electron? (Given charge of the electron = 1.6 x 10-19 C.)
The angle made by orbital angular momentum of electron with the direction of the orbital magnetic moment is ______.
An electron revolving in a circular orbit of radius 'r' with velocity 'v' and frequency v has orbital magnetic moment 'M'. If the frequency of revolution is tripled then the new magnetic moment will be ____________.
The electron in the hydrogen atom is moving with a speed of 1.5 x 106 m/s in an orbit of radius 2 Å. Magnetic moment of the revolving electron is ____________.
In a hydrogen atom, an electron of charge e revolves in a orbit of radius r with speed v. Then, the magnetic moment associated with electron is ______.
Which one of the following proportionality represents the relation between orbital magnetic moment M0 and orbital angular momentum L0 of an electron?
An electron revolving in a circular orbit of radius 'r' with velocity 'V' and frequency 'v' has orbital magnetic moment 'M'. If the frequency of revolution is doubled then the new magnetic moment will be ____________.
An electron (e) is revolving in a circular orbit of radius 'r' in hydrogen atom. The angular momentum of the electron is (M = magnetic dipole moment associated with it and m = mass of electron) ____________.
An electron of charge 'e' is revolving in a fixed orbit of radius 'r' with frequency 'f'. Its magnetic dipole moment is ____________.
An electron in the ground state of hydrogen atom is revolving in a circular orbit of radius R. The orbital magnetic moment of the electron is ____________. (m = mass of electron, h = Planck's constant, e = electronic charge)
In an atom, electron of charge (-e) perform U.C.M. around a stationary positively charged nucleus, with period of revolution 'T'. If 'r' is the radius of the orbit of the electron and 'v' is the orbital velocity, then the circulating current (I) is proportional to ____________.
The period of oscillation of a magnet in a vibration magnetometer is 2 sec. The period of oscillation of a magnet whose magnetic moment is four times that of the first magnet is ______.
Which of the following expression represents the relation between orbital magnetic moment and orbital angular momentum of an electron?
A bar magnet of magnetic moment M1 is cut into two pieces along its axis. The pieces are kept perpendicular to each other with their unlike poles in contact. The magnetic moment of the arrangement is M2. The ratio of M1/M2 is ______.
An electron in an atom is revolving around the nucleus in a circular orbit of radius 0.53 A, with a speed of 2 x 106 m/s. Find the resultant orbital magnetic moment and angular momentum of electron.(e = 1.6 x 10-19 C, m = 9.1 x 10-31 kg)
The electron in the hydrogen atom is moving with a speed at 2.3 x 106 m/s in an orbit of radius 0.53Å. Calculate the magnetic moment of the revolving electron.
