Advertisements
Advertisements
Question
Area of an isosceles triangle is 48 cm2. If the altitudes corresponding to the base of the triangle is 8 cm, find the perimeter of the triangle.
Advertisements
Solution
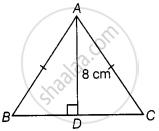
Given, area of ΔABC = 48 cm2 and altitude = 8 cm
∵ ΔABC is an isosceles triangle, where AB = AC
∴ Area of ΔABC = `1/2` × BC × AD = 48 ...[∵ Area of triangle = Base × Height]
⇒ 48 = `1/2` × BC × AD
⇒ `1/2` × BC × 8 = 48
⇒ BC = `(48 xx 2)/8`
BC = 12 cm
Now, in an isosceles triangle, BD = DC = 6 cm ...[∵ AD ⊥ BC]
Applying Pythagoras theorem in right-angled ΔADB,
AB2 = BD2 + AD2
⇒ AB2 = 62 + 82 = 36 + 64
⇒ AB2 = 100
⇒ AB = 10 cm
Now, perimeter of triangle = AB + AC + BC
= AB + AB + BC ...[∵ AB = AC]
= 10 + 10 + 12
= 32 cm
APPEARS IN
RELATED QUESTIONS
Find the area of a triangle whose sides are 18 cm, 24 cm, and 30 cm. Also, find the length of altitude corresponding to the largest side of the triangle.
The length of the sides of a triangle are in the ratio 3: 4: 5. Find the area of the triangle if its perimeter is 144 cm.
ABC is a triangle in which AB = AC = 4 cm and ∠A = 90°. Calculate:
(i) The area of ΔABC,
(ii) The length of the perpendicular from A to BC.
The base of a triangular field is three times its height. If the cost of cultivating the field at ₹ 36.72 per 100 m2 is ₹ 49,572; find its base and height.
The given figure shows a right-angled triangle ABC and an equilateral triangle BCD. Find the area of the shaded portion.
Find the area of a triangle, whose sides are :
10 cm, 24 cm, and 26 cm
The base and the height of a triangle are in the ratio 5 : 3. If the area of the triangle is 67.5 m2; find its base and height.
One of the equal sides of an isosceles triangle is 13 cm and its perimeter is 50 cm. Find the area of the triangle.
Find the area of a triangle whose sides are 27 cm, 45 cm and 36 cm.
The perimeter of a triangle is 72cm and its sides are in the ratio 3:4:5. Find its area and the length of the altitude corresponding to the longest side.
