Advertisements
Advertisements
Question
Attempts to prove Euclid’s fifth postulate using the other postulates and axioms led to the discovery of several other geometries.
Options
True
False
Solution
This statement is True.
Explanation:
All attempts to prove the fifth postulate as a theorem led to a great achievement in the creation of several other geometries. These geometries are quite different from Euclidean geometry and called non-Euclidean geometry.
APPEARS IN
RELATED QUESTIONS
Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’? (Note that the question is not about the fifth postulate.)
How many least number of distinct points determine a unique line?
Given three distinct points in a plane, how many lines can be drawn by joining them?
How many planes can be made to pass through a line and a point not on the line?
A pyramid is a solid figure, the base of which is ______.
Thales belongs to the country ______.
The edges of a surface are curves.
The statements that are proved are called axioms.
Solve the following question using appropriate Euclid’s axiom:
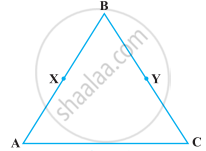
In the following figure, we have BX = `1/2` AB, BY = `1/2` BC and AB = BC. Show that BX = BY.
The following statement is true or false? Give reason for your answer.
If two circles are equal, then their radii are equal.
