Advertisements
Advertisements
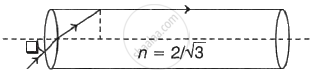
Question
Solution
Critical angle is the angle of incidence in the denser medium for which the angle of refraction in the rarer medium is 90o.
Total internal reflection: When a ray of light travelling from an optically denser medium to an optically rare medium is incident at an angle greater than the critical angle for the pair of media in contact, the ray is totally reflected back into the denser medium. This phenomenon is referred as total internal reflection.
The two necessary conditions for total internal reflection to take place are:
1. The light ray must proceed from denser to rarer medium.
2. Angle of incidence in denser medium should be greater than the critical angle for the pair of media in contact.
Relation between critical angle and r.i.: The critical angle can thus be calculated by taking the inverse-sine ratio of speed of light in denser medium and the speed of light in rarer.
ic = sin -1 `(("n"gamma)/("n"_i))`
APPEARS IN
RELATED QUESTIONS
What is total internal reflection?
A ray of light incident at an angle of incidence ‘i’ passes through an equilateral glass prism such that the refracted ray inside the prism is parallel to its base and emerges from the prism at an angle of emergence ‘e’.
What can you say about the value of the angle of deviation in such a situation?
Choose the correct option.
Select the WRONG statement.
Answer the following question.
Define the critical angle of incidence and obtain an expression for it.
Answer the following question.
Describe the construction and working of an optical fibre.
Solve Numerical example.
A monochromatic ray of light is incident at 37° on an equilateral prism of refractive index 3/2. Determine angle of emergence and angle of deviation. If angle of prism is adjustable, what should its value be for emergent rays to be just possible for the same angle of incidence?
The twinkling effect of star light is due to ______.
What are the conditions to achieve total internal reflection?
A ray of light passes through a prism of refractive index `sqrt2` as shown in the figure. Find:
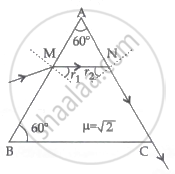
- The angle of incidence (∠r2) at face AC.
- The angle of minimum deviation for this prism.
Find the value of θ in the given figure.