Advertisements
Advertisements
Question
A spherical capacitor has an inner sphere of radius 12 cm and an outer sphere of radius 13 cm. The outer sphere is earthed and the inner sphere is given a charge of 2.5 µC. The space between the concentric spheres is filled with a liquid of dielectric constant 32.
(a) Determine the capacitance of the capacitor.
(b) What is the potential of the inner sphere?
(c) Compare the capacitance of this capacitor with that of an isolated sphere of radius 12 cm. Explain why the latter is much smaller.
Solution
Radius of the inner sphere, r2 = 12 cm = 0.12 m
Radius of the outer sphere, r1 = 13 cm = 0.13 m
Charge on the inner sphere, q = 2.5 µC = `2.5 xx 10^-6 "C"`
Dielectric constant of a liquid, ` in_"r" = 32`
(a) Capacitance of the capacitor is given by the relation
C = `(4piin_0in_1"r"_1"r"_2)/("r"_1 - "r"_2)`
Where,
`in_0` = Permittivity of free space = `8.85 xx 10^-12 "C"^2 "N"^-1 "m"^-2`
`1/(4piin_0) = 9 xx 10^9 "Nm"^2 "C"^-2`
∴ C = `(32 xx 0.12 xx 0.13)/(9 xx 10^9 xx (0.13 - 0.12))`
`≈ 5.5 xx 10^-9 "F"`
Hence, the capacitance of the capacitor is approximate `5.5 xx 10^-9 "F"`.
(b) Potential of the inner sphere is given by,
`"V" = "q"/"C"`
= `(2.5 xx 10^-6)/(5.5 xx 10^-9) = 4.5 xx 10^2 "V"`
Hence, the potential of the inner sphere is `4.5 xx 10^2 "V"`.
(c) Radius of an isolated sphere, r = 12 × 10−2 m
Capacitance of the sphere is given by the relation,
`"C'" = 4piin_0"r"`
= `4pi xx 8.85 xx 10^-12 xx 12 xx 10^-12`
= `1.33 xx 10^-11 "F"`
The capacitance of the isolated sphere is less in comparison to the concentric spheres. This is because the outer sphere of the concentric spheres is earthed. Hence, the potential difference is less and the capacitance is more than the isolated sphere.
APPEARS IN
RELATED QUESTIONS
A parallel plate capacitor of capacitance C is charged to a potential V. It is then connected to another uncharged capacitor having the same capacitance. Find out the ratio of the energy stored in the combined system to that stored initially in the single capacitor.
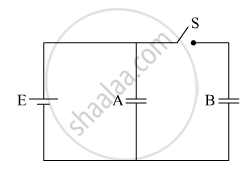
Two identical parallel plate capacitors A and B are connected to a battery of V volts with the switch S closed. The switch is now opened and the free space between the plates of the capacitors is filled with a dielectric of dielectric constant K. Find the ratio of the total electrostatic energy stored in both capacitors before and after the introduction of the dielectric.
A capacitor of unknown capacitance is connected across a battery of V volts. The charge stored in it is 360 μC. When potential across the capacitor is reduced by 120 V, the charge stored in it becomes 120 μC.
Calculate:
(i) The potential V and the unknown capacitance C.
(ii) What will be the charge stored in the capacitor, if the voltage applied had increased by 120 V?
A capacitor of unknown capacitance is connected across a battery of V volts. The charge stored in it is 300 μC. When potential across the capacitor is reduced by 100 V, the charge stored in it becomes 100 μC. Calculate The potential V and the unknown capacitance. What will be the charge stored in the capacitor if the voltage applied had increased by 100 V?
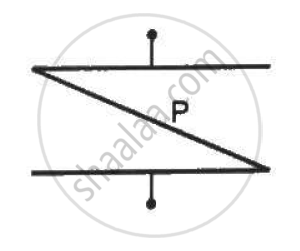
A thin metal plate P is inserted between the plates of a parallel-plate capacitor of capacitance C in such a way that its edges touch the two plates . The capacitance now becomes _________ .
Each of the plates shown in figure has surface area `(96/∈_0) xx 10^-12` Fm on one side and the separation between the consecutive plates is 4⋅0 mm. The emf of the battery connected is 10 volts. Find the magnitude of the charge supplied by the battery to each of the plates connected to it.

The separation between the plates of a parallel-plate capacitor is 0⋅500 cm and its plate area is 100 cm2. A 0⋅400 cm thick metal plate is inserted into the gap with its faces parallel to the plates. Show that the capacitance of the assembly is independent of the position of the metal plate within the gap and find its value.
Three capacitors C1 = 3μF, C2 = 6μF, and C3 = 10μF are connected to a 50 V battery as shown in Figure below:

Calculate:
(i) The equivalent capacitance of the circuit between points A and B.
(ii) The charge on C1.
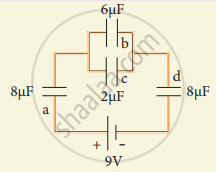
For the given capacitor configuration
- Find the charges on each capacitor
- potential difference across them
- energy stored in each capacitor.
The positive terminal of 12 V battery is connected to the ground. Then the negative terminal will be at ______.
The work done in placing a charge of 8 × 10–18 coulomb on a condenser of capacity 100 micro-farad is ______.
The radius of a sphere of capacity 1 microfarad in the air is ______
Can the potential function have a maximum or minimum in free space?
A parallel plate capacitor is filled by a dielectric whose relative permittivity varies with the applied voltage (U) as ε = αU where α = 2V–1. A similar capacitor with no dielectric is charged to U0 = 78V. It is then connected to the uncharged capacitor with the dielectric. Find the final voltage on the capacitors.
A capacitor of capacity 2 µF is charged to a potential difference of 12 V. It is then connected across an inductor of inductance 0.6 mH. The current in the circuit at a time when the potential difference across the capacitor is 6.0 V is ______ × 10-1A.
Read the following paragraph and answer the questions.
| A capacitor is a system of two conductors separated by an insulator. The two conductors have equal and opposite charges with a potential difference between them. The capacitance of a capacitor depends on the geometrical configuration (shape, size and separation) of the system and also on the nature of the insulator separating the two conductors. They are used to store charges. Like resistors, capacitors can be arranged in series or parallel or a combination of both to obtain the desired value of capacitance. |
- Find the equivalent capacitance between points A and B in the given diagram.

- A dielectric slab is inserted between the plates of the parallel plate capacitor. The electric field between the plates decreases. Explain.
- A capacitor A of capacitance C, having charge Q is connected across another uncharged capacitor B of capacitance 2C. Find an expression for (a) the potential difference across the combination and (b) the charge lost by capacitor A.
OR
Two slabs of dielectric constants 2K and K fill the space between the plates of a parallel plate capacitor of plate area A and plate separation d as shown in the figure. Find an expression for the capacitance of the system.
