Advertisements
Advertisements
Question
Draw a circle of radius 32 cm. Draw a tangent to the circle making an angle 30° with a line passing through the centre.
Solution
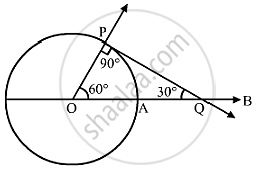
Steps Of construction:
Step 1: Draw a circle with center O and radius 3 cm.
Step 2: Draw radius OA and produce it to B.
Step 3: Make ∠AOP = 60°
Step 4: Draw PQ ⊥ OP,meeting OB at Q.
Step 5: Then, PQ is the desired tangent, such that ∠OQP = 30°
APPEARS IN
RELATED QUESTIONS
Draw a line segment AB of length 8 cm. Taking A as centre, draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Construct tangents to each circle from the centre of the other circle.
Draw a circle with the help of a bangle. Take a point outside the circle. Construct the pair of tangents from this point to the circles. Give the justification of the construction.
Draw a circle of radius 5 cm. Draw two tangents to this circle so that the angle between the tangents is 45°.
- Using ruler and compasses only, construct a triangle ABC in which AB = 8 cm, BC = 6 cm and CA = 5 cm.
- Find its in centre and mark it I.
- With I as centre, draw a circle which will cut off 2 cm chords from each side of the triangle. What is the length of the radius of this circle.
Draw a circle circumscribing a regular hexagon with side 5 cm.
Write the steps of construction for drawing a pair of tangents to a circle of radius 3 cm , which are inclined to each other at an angle of 60° .
Draw a circle with centre O and radius 3 cm. Take a point P outside the circle. Draw tangents to the circle from P without using the centre and using only ruler and compasses.
Use a ruler and a pair of compasses to construct ΔABC in which BC = 4.2 cm, ∠ ABC = 60°, and AB 5 cm. Construct a circle of radius 2 cm to touch both the arms of ∠ ABC of Δ ABC.
To draw a pair of tangents to a circle which are inclined to each other at an angle of 60°, it is required to draw tangents at end points of those two radii of the circle, the angle between them should be ______.
Which of the following is not true for a point P on the circle?
