Advertisements
Advertisements
Question
Draw the tangent at any point M on the circle with centre O and radius 2.9 cm.
Solution
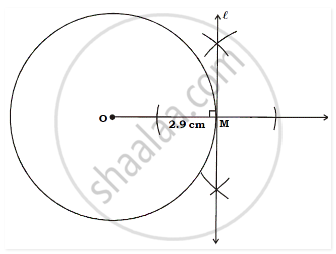
For drawing the circle of radius 2.9 cm extending the line through M Drawing perpendicular at point M.
APPEARS IN
RELATED QUESTIONS
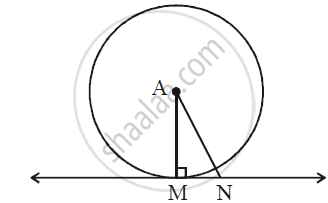
In the following figure, point ‘A’ is the centre of the circle. Line MN is tangent at point M. If AN = 10 cm and MN = 5 cm, determine radius of the circle.
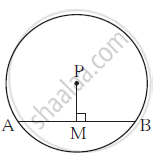
P is the centre of the circle and its radius is
10 cm. Distance of a chord AB from the centre is 6 cm. Find the length of chord AB.
Radius of circular base of an ear of corn is 6.6 cm and its length is
11.2 cm. If on an average 1 sqcm area contains 2 corn kernels, find the
total number of kernels on a corn.
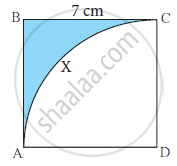
Side of square ABCD is 7 cm. With D as the centre and DA as radius, arc AXC is drawn.Find the area of the shaded region with the help of the following flow chart .

There is a hemispherical bowl. A cone is to be made such that, if it is filled with water twice and the water is poured in the bowl, it will be filled just completely. State how will you decide the radius and perpendicular height of the cone.
In the figure, line l touches the circle with center O at point P. Q is the midpoint of radius OP. RS is a chord through Q such that chords RS || line l. If RS = 12, find the radius of the circle.

In the given figure, seg AB is a diameter of a circle with centre C. Line PQ is a tangent, which touches the circle at point T. seg AP ⊥ line PQ and seg BQ ⊥ line PQ. Prove that, seg CP ≅ seg CQ.

Draw a tangent at any point ‘M’ on the circle of radius 3.3 cm and centre ‘O’.
In the adjoining figure the radius of a circle with centre C is 6 cm, line AB is a tangent at A. What is the measure of ∠CAB? Why?

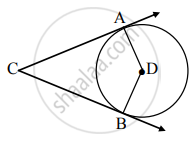
In the adjoining figure, circle with center D touches the sides of ∠ACB at A and B. If ∠ACB = 52°, find measure of ∠ADB.
In the adjoining figure, the line MN touches the circle with center A at point M. If AN = 13 and MN = 5, then find the radius of the circle?

Draw a circle with centre O having radius 3 cm. Draw tangent segments PA and PB through the point P outside the circle such that ∠APB = 70°.
