Advertisements
Advertisements
Question
Evaluate: `cos (sin^-1 (4/5) + sin^-1 (12/13))`
Solution
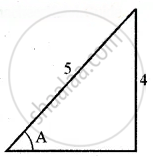
`sqrt(5^2 - 4^2)` = 3
cos A = `"Adj"/"Hyp" = 3/5`
Let `sin^-1 (4/5)` = A
sin A = `4/5`
∴ cos A = `3/5`
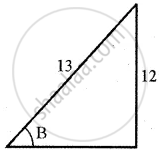
`sqrt(169 - 144) = sqrt 25` = 5
cos B = `"Adj"/"Hyp" = 5/13`
Let `sin^-1 (12/13)` = B
`12/13` = sin B
sin B = `12/13`
∴ cos B = `5/13`
Now `cos (sin^-1 (4/5) + sin^-1 (12/13))` = cos (A + B)
= cos A cos B – sin A sin B
`= 3/5 xx 5/13 - 4/5 xx 12/13`
`= 15/65 - 48/65`
`= - 33/65`
APPEARS IN
RELATED QUESTIONS
Find the principal value of `sin^-1(1/sqrt2)`
In ΔABC, if a = 18, b = 24, c = 30 then find the values of cos `A/2`
Find the principal value of the following: tan- 1( - √3)
When `"x" = "x"/2`, then tan x is ____________.
If `"x" in (- pi/2, pi/2), "then the value of tan"^-1 ("tan x"/4) + "tan"^-1 ((3 "sin" 2 "x")/(5 + 3 "cos" 2 "x"))` is ____________.
`2"tan"^-1 ("cos x") = "tan"^-1 (2 "cosec x")`
The equation of the tangent to the curve given by x = a sin3t, y = bcos3t at a point where t = `pi/2` is
Assertion (A): The domain of the function sec–12x is `(-∞, - 1/2] ∪ pi/2, ∞)`
Reason (R): sec–1(–2) = `- pi/4`
Let x = sin–1(sin8) + cos–1(cos11) + tan–1(tan7), and x = k(π – 2.4) for an integer k, then the value of k is ______.
Find the value of `tan^-1(x/y) + tan^-1((y - x)/(y + x))`
