Question

Find the area of the triangle PQR with Q(3,2) and the mid-points of the sides through Q being (2,−1) and (1,2).

Answer 1

Let the coordinates of the vertices P and R of ∆PQR be (a_1, b₁) and (a_2, b₂), respectively.

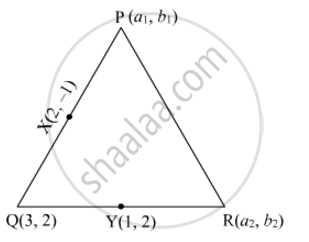
Suppose X(2, −1) is the midpoint of PQ.

Then,

`(2,-1)=((a_1+3)/2, (b_1+2)/2)`

`=>(a_1+3)/2=2 `

⇒ a₁=1 and b₁=−4

Therefore, the coordinates of P are (1, −4).

Again, suppose Y(1, 2) is the midpoint of QR.

Now,

`(1,2)=((a_2+3)/2,(b_2+2)/2)`

`=>(a_2+3)/2=1 `

⇒ a₂=−1 and b₂=2

Therefore, the coordinates of R are (−1, 2).

Thus, the vertices of ∆PQR are P(1,−4), Q(3, 2) and R(−1, 2).

Now,

Area of ∆PQR =`1/2`×[1(2−2)+3(2+4)−1(−4−2)]

=`1/2`(18+6)

=`1/2`(24)

=12

Thus, the area of ∆PQR is 12 square units.