Advertisements
Advertisements
Question
If Δ = `|(1, x, x^2),(1, y, y^2),(1, z, z^2)|`, Δ1 = `|(1, 1, 1),(yz, zx, xy),(x, y, z)|`, then prove that ∆ + ∆1 = 0.
Solution
We have Δ1 = `|(1, 1, 1),(yz, zx, xy),(x, y, z)|`
Interchanging rows and columns, we get
Δ1 = `|(1, yz, x),(1, z, y),(1, xy, z)|`
= `1/(xyz) |(x, xyz, x^2),(y, xyz, y^2),(z, xyz, z^2)|`
= `(xyz)/(xyz) |(x, 1, x^2),(y, 1, y^2),(z, 1, z^2)|`
Interchanging C1 and C2
= `(-1)|(1, x, x^2),(1, y, y^2),(1, z, z^2)|`
= – Δ
⇒ Δ1 + Δ = 0
APPEARS IN
RELATED QUESTIONS
Find the values of k for which the points A(k + 1, 2k), B(3k, 2k + 3) and (5k – 1, 5k) are collinear.
If P(–5, –3), Q(–4, –6), R(2, –3) and S(1, 2) are the vertices of a quadrilateral PQRS, find its area.
Find the area of a triangle whose vertices are A(3, 2), B (11, 8) and C(8, 12).
Find the area of the quadrilateral ABCD whose vertices are respectively A(1, 1), B(7, –3), C(12, 2) and D(7, 21).
Find the area of a triangle with vertices at the point given in the following:
(1, 0), (6, 0), (4, 3)
ΔABC is isosceles with AB = AC = 7.5 cm and BC = 9 cm (see the given figure). The height AD from A to BC, is 6 cm. Find the area of ΔABC. What will be the height from C to AB i.e., CE?

If A(–5, 7), B(–4, –5), C(–1, –6) and D(4, 5) are the vertices of a quadrilateral, find the area of the quadrilateral ABCD
Prove that the points (2a, 4a), (2a, 6a) and `(2a + sqrt3a, 5a)` are the vertices of an equilateral triangle.
The point A divides the join of P (−5, 1) and Q(3, 5) in the ratio k:1. Find the two values of k for which the area of ΔABC where B is (1, 5) and C(7, −2) is equal to 2 units.
Find the centroid of the triangle whosw vertices is (1,4), (-1,1) and (3,2) .
In a ΔABC, AB = 15 cm, BC = 13 cm and AC = 14 cm. Find the area of ΔABC and hence its altitude on AC ?
If A(5,2), B(2, -2) and C(-2, t) are the vertices of a right triangle with ∠B=90° , then find the value of t .
For what value of k(k>0) is the area of the triangle with vertices (-2, 5), (k, -4) and (2k+1, 10) equal to 53 square units?
Show that the following points are collinear:
(i) A(2,-2), B(-3, 8) and C(-1, 4)
If the points P(-3, 9), Q(a, b) and R(4, -5) are collinear and a+b=1, find the value of a and b.
Find the value of x for which the points (x, −1), (2, 1) and (4, 5) are collinear ?
Find the area of the following triangle:

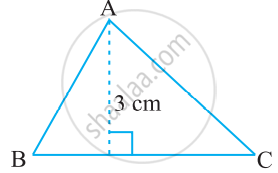
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
The area of a triangle with vertices (–3, 0), (3, 0) and (0, k) is 9 sq.units. The value of k will be ______.
If the points (3, -2), (x, 2), (8, 8) are collinear, then find the value of x.
Let `Delta = abs (("x", "y", "z"),("x"^2, "y"^2, "z"^2),("x"^3, "y"^3, "z"^3)),` then the value of `Delta` is ____________.
If the points A(1, 2), O(0, 0) and C(a, b) are collinear, then ______.
The area of a triangle with base 4 cm and height 6 cm is 24 cm2.
The area of ∆ABC is 8 cm2 in which AB = AC = 4 cm and ∠A = 90º.
The base and the corresponding altitude of a parallelogram are 10 cm and 3.5 cm, respectively. The area of the parallelogram is 30 cm2.
The area of a triangle with vertices A, B, C is given by ______.
In the given figure, if PR = 12 cm, QR = 6 cm and PL = 8 cm, then QM is ______.

Ratio of the area of ∆WXY to the area of ∆WZY is 3:4 in the given figure. If the area of ∆WXZ is 56 cm2 and WY = 8 cm, find the lengths of XY and YZ.

Find the missing value:
| Base | Height | Area of Triangle |
| ______ | 31.4 mm | 1256 mm2 |
