Advertisements
Advertisements
Question
The area of a triangle with vertices (–3, 0), (3, 0) and (0, k) is 9 sq.units. The value of k will be ______.
Options
9
±3
– 9
6
Solution
The area of a triangle with vertices (–3, 0), (3, 0) and (0, k) is 9 sq.units. The value of k will be ±3.
Explanation:
We know that, area of triangle with vertices (x1, y1), (x2, y2) and (x3, y3) is given by
Δ = `1/2|(x_1, y_1, 1),(x_2, y_2, 1),(x_3, y_3, 1)|`
∴ Area of triangle with verticles (–3, 0), (3, 0) and (0, k) is
∴ Δ = `1/2|(-3, 0, 1),(3, 0, 1),(0, "k", 1)|` = 9 ...(Given)
⇒ `[-3(-"k") - 0 + 1(3"k")]` = ±18
⇒ 6k = ±18
∴ k = `+- 18/6` = ±3
RELATED QUESTIONS
Prove that the area of a triangle with vertices (t, t −2), (t + 2, t + 2) and (t + 3, t) is independent of t.
If the points A(−2, 1), B(a, b) and C(4, −1) are collinear and a − b = 1, find the values of a and b.
If A(−4, 8), B(−3, −4), C(0, −5) and D(5, 6) are the vertices of a quadrilateral ABCD, find its area.
Prove that the points (a, b + c), (b, c + a) and (c, a + b) are collinear
The coordinates of A, B, C are (6, 3), (–3, 5) and (4, – 2) respectively and P is any point (x, y). Show that the ratio of the areas of triangle PBC and ABC is
Find the area of the quadrilateral whose vertices, taken in order, are (-4, -2), (-3, -5), (3, -2) and (2, 3).
Determine the ratio in which the line 2x + y – 4 = 0 divides the line segment joining the points A(2, – 2) and B(3, 7).
Find the area of a triangle with vertices at the point given in the following:
(1, 0), (6, 0), (4, 3)
The area of a triangle is 5 sq units. Two of its vertices are (2, 1) and (3, –2). If the third vertex is (`7/2`, y). Find the value of y
Show that the following sets of points are collinear.
(1, −1), (2, 1) and (4, 5)
The point A divides the join of P (−5, 1) and Q(3, 5) in the ratio k:1. Find the two values of k for which the area of ΔABC where B is (1, 5) and C(7, −2) is equal to 2 units.
Two vertices of a triangle are (1, 2), (3, 5) and its centroid is at the origin. Find the coordinates of the third vertex.
Prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another
In a ΔABC, AB = 15 cm, BC = 13 cm and AC = 14 cm. Find the area of ΔABC and hence its altitude on AC ?
If A(5,2), B(2, -2) and C(-2, t) are the vertices of a right triangle with ∠B=90° , then find the value of t .
Prove that the points A(2, 4), b(2, 6) and (2 +`sqrt(3)` ,5) are the vertices of an equilateral triangle
Find the third vertex of a ΔABC if two of its vertices are B(-3,1) and C (0,-2) and its centroid is at the origin
Find the area of ΔABC whose vertices are:
A(10,-6) , B (2,5) and C(-1,-3)
Find the value(s) of k so that the quadratic equation x2 − 4kx + k = 0 has equal roots.
Using integration, find the area of triangle ABC, whose vertices are A(2, 5), B(4, 7) and C(6, 2).
Find the area of the following triangle:

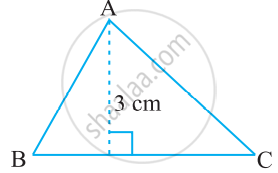
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
The value of the determinant `abs((1,"x","x"^3),(1,"y","y"^3),(1,"z","z"^3))` is ____________.
If the points (a1, b1), (a2, b2) and(a1 + a2, b1 + b2) are collinear, then ____________.
The points (0, 5), (0, –9) and (3, 6) are collinear.
Points A(–6, 10), B(–4, 6) and C(3, –8) are collinear such that AB = `2/9` AC.
Find the area of the triangle whose vertices are (–8, 4), (–6, 6) and (–3, 9).
The base and the corresponding altitude of a parallelogram are 10 cm and 3.5 cm, respectively. The area of the parallelogram is 30 cm2.
A rectangular plot is given for constructing a house, having a measurement of 40 m long and 15 m in the front. According to the laws, a minimum of 3 m, wide space should be left in the front and back each and 2 m wide space on each of other sides. Find the largest area where house can be constructed.
Ratio of the area of ∆WXY to the area of ∆WZY is 3:4 in the given figure. If the area of ∆WXZ is 56 cm2 and WY = 8 cm, find the lengths of XY and YZ.

