Advertisements
Advertisements
Question
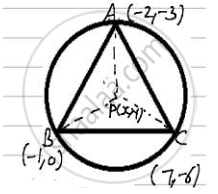
Find the circumcenter of the triangle whose vertices are (-2, -3), (-1, 0), (7, -6).
Advertisements
Solution
The circumference of a triangle is equidistance from the vertices of a triangle.
Let A(-2, -3), B(-1, 0) and C(7, -6) vertices of the given triangle and let P(x,y) be the circumference of this triangle, Then
PA = PB = PC
Now, PA = PB
`=> sqrt((-2-x)^2 + (-3 -y)^2) = sqrt((-1 - x)^2 + (0 - y)^2`
`=> 4 + x^2 + 4x + 9 + y^2 + 6y = 1 + x^2 + 2xz + y^2`
`=> 4 + x^2 + 4x + 9 + y^2 + 6y - 1 - x^2 - 2x - y^2 = 0`
`=> 2x + 6y + 12 = 0
=> 2(x + 3y + 6) = 0
=> x + 3y + 6 = 0 ... eq (1)
And PB = PC
`=> sqrt((-1-x)^2 + (0 - y)^2) = sqrt((7- x)^2 + (-6 - y)^2)`
Squaring both the sides
`=> (-1 - x)^2 + y^2 = (7 - x)^2 + (-6 -y)^2`
`=> 1 + x^2 + 2x + y^2 = (7 - x)^2 + (-6 - y)^2`
`=> 1 + x^2 + 2x + y^2 - 49 - x^2 + 14x - 36 - y^2 - 12y`
=> 16x - 12y - 84 = 0
`=> 4(4x - 3y - 21) = 0`
=> 4x - 3y - 21 = 0 ......eq(2)
Adding eq(1) and eq(2)
`=> x + 3y + 6 + 4x - 3y - 21 = 0`
=> x + 3y + 6 + 4x -3y - 21 = 0
=> 5x - 15 = 0
=> x = 15/5`
=> x = 3
putting the value of x in eq (2) and
we get
`=> 4 x 3 - 3y - 21 = 0`
`=> 12 - 3y - 21 = 0`
`=> -3y - 9 = 0`
`=> y = (-9)/3 = -3`
So the coordinates of the circumcentre P are (3, -3)
RELATED QUESTIONS
Find the value of x, if the distance between the points (x, – 1) and (3, 2) is 5.
Name the type of quadrilateral formed, if any, by the following point, and give reasons for your answer:
(4, 5), (7, 6), (4, 3), (1, 2)
Find the point on the x-axis which is equidistant from (2, -5) and (-2, 9).
Find the distance between the following pair of points:
(-6, 7) and (-1, -5)
Find the value of a when the distance between the points (3, a) and (4, 1) is `sqrt10`
An equilateral triangle has two vertices at the points (3, 4) and (−2, 3), find the coordinates of the third vertex.
Find the distance between the points
(i) A(9,3) and B(15,11)
P(5 , -8) , Q (2 , -9) and R(2 , 1) are the vertices of a triangle. Find tyhe circumcentre and the circumradius of the triangle.
Prove that the points (6 , -1) , (5 , 8) and (1 , 3) are the vertices of an isosceles triangle.
A(2, 5), B(-2, 4) and C(-2, 6) are the vertices of a triangle ABC. Prove that ABC is an isosceles triangle.
Find the distance between the points (a, b) and (−a, −b).
In what ratio does the point P(−4, y) divides the line segment joining the points A(−6, 10) and B(3, −8)? Hence find the value of y.
Find the co-ordinates of points on the x-axis which are at a distance of 17 units from the point (11, -8).
A point P lies on the x-axis and another point Q lies on the y-axis.
Write the ordinate of point P.
If the distance between point L(x, 7) and point M(1, 15) is 10, then find the value of x
Show that P(– 2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle
The distance between the points (0, 5) and (–5, 0) is ______.
The distance between the point P(1, 4) and Q(4, 0) is ______.
Name the type of triangle formed by the points A(–5, 6), B(–4, –2) and C(7, 5).
A point (x, y) is at a distance of 5 units from the origin. How many such points lie in the third quadrant?
