Advertisements
Advertisements
Question
Find the coordinates of the point which divides the line segment joining (−1,3) and (4, −7) internally in the ratio 3 : 4
Solution
We have A (−1, 3) and B (4,−7) be two points. Let a point P(x, y) divide the line segment joining the points A and B in the ratio 3:4 internally.
Now according to the section formula if point a point P divides a line segment joining `A(x_1, y_1)` and B`(x_2, y_2)` in the ratio m: n internally than,
P(x,y) = ((nx_1 + mx_2)/(m+n), (ny_1 + my_2)/(m+n))
`= (8/7,-9/7)`
Therefore, co-ordinates of point P is `(8/7,-9/7)`
APPEARS IN
RELATED QUESTIONS
If two opposite vertices of a square are (5, 4) and (1, −6), find the coordinates of its remaining two vertices.
If G be the centroid of a triangle ABC, prove that:
AB2 + BC2 + CA2 = 3 (GA2 + GB2 + GC2)
In Fig. 14.36, a right triangle BOA is given C is the mid-point of the hypotenuse AB. Show that it is equidistant from the vertices O, A and B.

We have a right angled triangle,`triangle BOA` right angled at O. Co-ordinates are B (0,2b); A (2a, 0) and C (0, 0).
Name the quadrilateral formed, if any, by the following points, and given reasons for your answers:
A(4, 5) B(7, 6), C (4, 3), D(1, 2)
The points A(2, 0), B(9, 1) C(11, 6) and D(4, 4) are the vertices of a quadrilateral ABCD. Determine whether ABCD is a rhombus or not.
Show that the following points are the vertices of a square:
A (6,2), B(2,1), C(1,5) and D(5,6)
Show that the following points are the vertices of a square:
A (0,-2), B(3,1), C(0,4) and D(-3,1)
In what ratio does the line x - y - 2 = 0 divide the line segment joining the points A (3, 1) and B (8, 9)?
Find the area of quadrilateral ABCD whose vertices are A(-5, 7), B(-4, -5) C(-1,-6) and D(4,5)
ABCD is a rectangle whose three vertices are A(4,0), C(4,3) and D(0,3). Find the length of one its diagonal.
Find the possible pairs of coordinates of the fourth vertex D of the parallelogram, if three of its vertices are A(5, 6), B(1, –2) and C(3, –2).
A point whose abscissa is −3 and ordinate 2 lies in
The area of the triangle formed by the points P (0, 1), Q (0, 5) and R (3, 4) is
If the point \[C \left( - 1, 2 \right)\] divides internally the line segment joining the points A (2, 5) and B( x, y ) in the ratio 3 : 4 , find the value of x2 + y2 .
If the point P (m, 3) lies on the line segment joining the points \[A\left( - \frac{2}{5}, 6 \right)\] and B (2, 8), find the value of m.
Which of the points P(-1, 1), Q(3, - 4), R(1, -1), S (-2, -3), T(-4, 4) lie in the fourth quadrant?
The line segment joining the points (3, -1) and (-6, 5) is trisected. The coordinates of point of trisection are ______.
The distance of the point (–6, 8) from x-axis is ______.
Ryan, from a very young age, was fascinated by the twinkling of stars and the vastness of space. He always dreamt of becoming an astronaut one day. So, he started to sketch his own rocket designs on the graph sheet. One such design is given below :
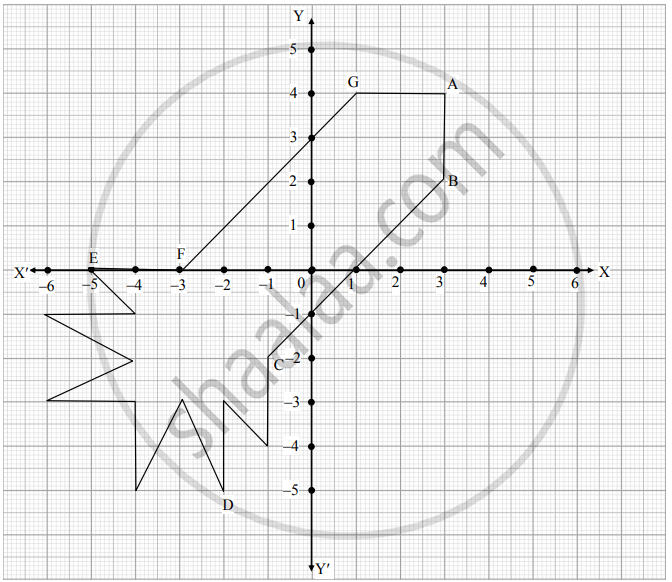
Based on the above, answer the following questions:
i. Find the mid-point of the segment joining F and G. (1)
ii. a. What is the distance between the points A and C? (2)
OR
b. Find the coordinates of the points which divides the line segment joining the points A and B in the ratio 1 : 3 internally. (2)
iii. What are the coordinates of the point D? (1)
