Advertisements
Advertisements
Question
Find the coordinates of the point which is equidistant from the three vertices A (\[2x, 0) O (0, 0) \text{ and } B(0, 2y) of ∆\] AOB .
Solution

It is known that, in a right angled triangle midpoint of the hypotenuse is equidistant from ots vertices.
Suppose D be the midpoint of the hypotenuse AB.
The coordinates of D are \[\left( \frac{2x + 0}{2}, \frac{0 + 2y}{2} \right) = \left( x, y \right)\] .
APPEARS IN
RELATED QUESTIONS
Find a point on the x-axis which is equidistant from the points (7, 6) and (−3, 4).
Show that the points A(5, 6), B(1, 5), C(2, 1) and D(6,2) are the vertices of a square.
Prove that the points (0, 0), (5, 5) and (-5, 5) are the vertices of a right isosceles triangle.
Find the points of trisection of the line segment joining the points:
(2, -2) and (-7, 4).
Find the coordinates of the point where the diagonals of the parallelogram formed by joining the points (-2, -1), (1, 0), (4, 3) and(1, 2) meet
Find the ratio in which the line segment joining (-2, -3) and (5, 6) is divided by x-axis Also, find the coordinates of the point of division in each case.
The line segment joining the points A(3,−4) and B(1,2) is trisected at the points P(p,−2) and Q `(5/3,q)`. Find the values of p and q.
Find the value of a, so that the point ( 3,a ) lies on the line represented by 2x - 3y =5 .
Find the coordinates of circumcentre and radius of circumcircle of ∆ABC if A(7, 1), B(3, 5) and C(2, 0) are given.
Find the coordinates of the centre of the circle passing through the points P(6, –6), Q(3, –7) and R (3, 3).
If `P(a/2,4)`is the mid-point of the line-segment joining the points A (−6, 5) and B(−2, 3), then the value of a is
Show that A(-4, -7), B(-1, 2), C(8, 5) and D(5, -4) are the vertices of a
rhombus ABCD.
Points (−4, 0) and (7, 0) lie
If \[D\left( - \frac{1}{5}, \frac{5}{2} \right), E(7, 3) \text{ and } F\left( \frac{7}{2}, \frac{7}{2} \right)\] are the mid-points of sides of \[∆ ABC\] , find the area of \[∆ ABC\] .
The coordinates of the fourth vertex of the rectangle formed by the points (0, 0), (2, 0), (0, 3) are
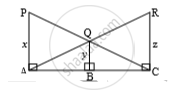
In the above figure, seg PA, seg QB and RC are perpendicular to seg AC. From the information given in the figure, prove that: `1/x + 1/y = 1/z`
The line segment joining the points (3, -1) and (-6, 5) is trisected. The coordinates of point of trisection are ______.
In which quadrant, does the abscissa, and ordinate of a point have the same sign?
If the points P(1, 2), Q(0, 0) and R(x, y) are collinear, then find the relation between x and y.
Given points are P(1, 2), Q(0, 0) and R(x, y).
The given points are collinear, so the area of the triangle formed by them is `square`.
∴ `1/2 |x_1(y_2 - y_3) + x_2(y_3 - y_1) + x_3(y_1 - y_2)| = square`
`1/2 |1(square) + 0(square) + x(square)| = square`
`square + square + square` = 0
`square + square` = 0
`square = square`
Hence, the relation between x and y is `square`.
Assertion (A): Mid-point of a line segment divides the line segment in the ratio 1 : 1
Reason (R): The ratio in which the point (−3, k) divides the line segment joining the points (− 5, 4) and (− 2, 3) is 1 : 2.
