Advertisements
Advertisements
Question
Find the measure of each angle of an equilateral triangle.
Solution
In equilateral triangle we know that each angle is equal
So ∠A = ∠B = ∠C
Now ∠A + ∠B + ∠C = 180° (by triangle property)
3∠A = 180°
∠A = 60°
Hence. ∠A = ∠B = ∠C = 60°
APPEARS IN
RELATED QUESTIONS
In a ΔABC, if AB = AC and BC is produced to D such that ∠ACD = 100°, then ∠A =
Which of the following is not a criterion for congruence of triangles?
In the pair of triangles in the following figure, parts bearing identical marks are congruent. State the test and the correspondence of vertices by the triangle in pairs is congruent.

On the sides AB and AC of triangle ABC, equilateral triangle ABD and ACE are drawn. Prove that:
- ∠CAD = ∠BAE
- CD = BE
The following figure shown a triangle ABC in which AB = AC. M is a point on AB and N is a point on AC such that BM = CN.
Prove that: ΔAMC≅ ΔANB

AD and BE are altitudes of an isosceles triangle ABC with AC = BC. Prove that AE = BD.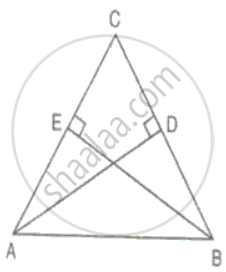
In ΔABC, AB = AC, BM and Cn are perpendiculars on AC and AB respectively. Prove that BM = CN.
If the given two triangles are congruent, then identify all the corresponding sides and also write the congruent angles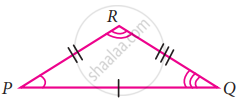
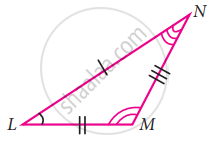
If ∆PQR is congruent to ∆STU (see figure), then what is the length of TU?
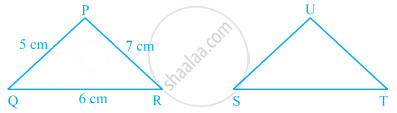
Without drawing the triangles write all six pairs of equal measures in the following pairs of congruent triangles.
∆ABC ≅ ∆LMN
