Advertisements
Chapters
2: Exponents of Real Numbers
3: Rationalisation
4: Algebraic Identities
5: Factorisation of Algebraic Expressions
6: Factorisation of Polynomials
7: Linear Equations in Two Variables
8: Co-ordinate Geometry
9: Introduction to Euclid’s Geometry
10: Lines and Angles
11: Triangle and its Angles
▶ 12: Congruent Triangles
13: Quadrilaterals
14: Areas of Parallelograms and Triangles
15: Circles
16: Constructions
17: Heron’s Formula
18: Surface Areas and Volume of a Cuboid and Cube
19: Surface Areas and Volume of a Circular Cylinder
20: Surface Areas and Volume of A Right Circular Cone
21: Surface Areas and Volume of a Sphere
22: Tabular Representation of Statistical Data
23: Graphical Representation of Statistical Data
24: Measures of Central Tendency
25: Probability
![RD Sharma solutions for Mathematics [English] Class 9 chapter 12 - Congruent Triangles RD Sharma solutions for Mathematics [English] Class 9 chapter 12 - Congruent Triangles - Shaalaa.com](/images/8193647912-mathematics-english-class-9_6:1a030933ece146238cec338f12706a07.jpg)
Advertisements
Solutions for Chapter 12: Congruent Triangles
Below listed, you can find solutions for Chapter 12 of CBSE RD Sharma for Mathematics [English] Class 9.
RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.1 [Pages 15 - 16]
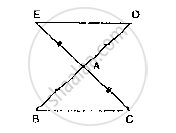
In Fig. 10.22, the sides BA and CA have been produced such that: BA = AD and CA = AE.
Prove that segment DE || BC.
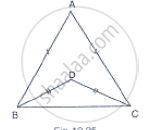
In a ΔPQR, if PQ = QR and L, M and N are the mid-points of the sides PQ, QR and RP
respectively. Prove that: LN = MN.
Prove that the medians of an equilateral triangle are equal.
In a ΔABC, if ∠A=l20° and AB = AC. Find ∠B and ∠C.
In a ΔABC, if AB = AC and ∠B = 70°, find ∠A.
The vertical angle of an isosceles triangle is 100°. Find its base angles.
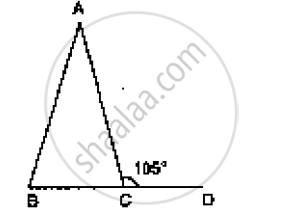
In Figure AB = AC and ∠ACD =105°, find ∠BAC.
Find the measure of each exterior angle of an equilateral triangle.
If the base of an isosceles triangle is produced on both sides, prove that the exterior angles so formed are equal to each other.
In figure, AB = AC and DB = DC, find the ratio ∠ABD : ∠ACD
Determine the measure of each of the equal angles of a right-angled isosceles triangle.
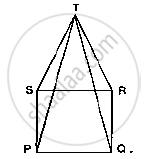
In Fig. 10.23, PQRS is a square and SRT is an equilateral triangle. Prove that
(i) PT = QT (ii) ∠TQR = 15°
AB is a line seg P and Q are points on opposite sides of AB such that each of them is equidistant from the points A and B (See Fig. 10.26). Show that the line PQ is perpendicular bisector of AB.
RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.2 [Page 25]
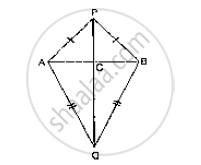
BD and CE are bisectors of ∠B and ∠C of an isosceles ΔABC with AB = AC. Prove that BD = CE.
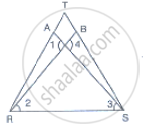
In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT
Two lines AB and CD intersect at O such that BC is equal and parallel to AD. Prove that the lines AB and CD bisect at O.
RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.3 [Page 47]
In two right triangles one side an acute angle of one are equal to the corresponding side and angle of the othe Prove that the triangles are congruent.
If the bisector of the exterior vertical angle of a triangle be parallel to the base. Show that the triangle is isosce
In an isosceles triangle, if the vertex angle is twice the sum of the base angles, calculate the angles of the triangle.
Prove that each angle of an equilateral triangle is 60°.
Angles A, B, C of a triangle ABC are equal to each other. Prove that ΔABC is equilateral.
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
PQR is a triangle in which PQ = PR and S is any point on the side PQ. Through S, a line is drawn parallel to QR and intersecting PR at T. Prove that PS = PT.
In a ΔABC, it is given that AB = AC and the bisectors of ∠B and ∠C intersect at O. If M is a point on BO produced, prove that ∠MOC = ∠ABC.
P is a point on the bisector of an angle ∠ABC. If the line through P parallel to AB meets BC at Q, prove that triangle BPQ is isosceles.
ABC is a triangle in which ∠B = 2 ∠C. D is a point on BC such that AD bisects ∠BAC and AB = CD.
Prove that ∠BAC = 72°.
RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.4 [Page 57]
In Fig. 10.92, it is given that AB = CD and AD = BC. Prove that ΔADC ≅ ΔCBA.

RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.5 [Pages 61 - 62]
ABC is a triangle and D is the mid-point of BC. The perpendiculars from D to AB and AC are equal. Prove that the triangle is isosceles.
ABC is a triangle in which BE and CF are, respectively, the perpendiculars to the sides AC and AB. If BE = CF, prove that ΔABC is isosceles
If perpendiculars from any point within an angle on its arms are congruent, prove that it lies on the bisector of that angle.
In Fig. 10.99, AD ⊥ CD and CB ⊥. CD. If AQ = BP and DP = CQ, prove that ∠DAQ = ∠CBP.

Which of the following statements are true (T) and which are false (F):
Sides opposite to equal angles of a triangle may be unequal
Which of the following statements are true (T) and which are false (F):
Angles opposite to equal sides of a triangle are equal
Which of the following statements are true (T) and which are false (F):
The measure of each angle of an equilateral triangle is 60°
Which of the following statements are true (T) and which are false (F) :
If the altitude from one vertex of a triangle bisects the opposite side, then the triangle may be isosceles.
Which of the following statements are true (T) and which are false (F):
The bisectors of two equal angles of a triangle are equal
Which of the following statements are true (T) and which are false (F):
If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles.
Which of the following statements are true (T) and which are false (F):
The two altitudes corresponding to two equal sides of a triangle need not be equal.
Which of the following statements are true (T) and which are false (F):
If any two sides of a right triangle are respectively equal to two sides of other right triangle, then the two triangles are congruent.
True
False
Which of the following statements are true (T) and which are false (F):
Two right triangles are congruent if hypotenuse and a side of one triangle are respectively equal equal to the hypotenuse and a side of the other triangle.
True
False
Fill the blank in the following so that the following statement is true.
Sides opposite to equal angles of a triangle are ......
Fill the blank in the following so that the following statement is true.
Angle opposite to equal sides of a triangle are .....
Fill the blank in the following so that the following statement is true.
In an equilateral triangle all angles are .....
Fill the blank in the following so that the following statement is true.
In a ΔABC if ∠A = ∠C , then AB = ......
Fill the blank in the following so that the following statement is true.
If altitudes CE and BF of a triangle ABC are equal, then AB = ....
Fill the blank in the following so that the following statement is true.
In an isosceles triangle ABC with AB = AC, if BD and CE are its altitudes, then BD is …… CE.
Fill the blank in the following so that the following statement is true.
In right triangles ABC and DEF, if hypotenuse AB = EF and side AC = DE, then ΔABC ≅ Δ ……
ABCD is a square, X and Yare points on sides AD and BC respectively such that AY = BX. Prove that BY = AX and ∠BAY = ∠ABX.
RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.6 [Pages 81 - 82]
In ΔABC, if ∠A = 40° and ∠B = 60°. Determine the longest and shortest sides of the triangle.
In a ΔABC, if ∠B = ∠C = 45°, which is the longest side?
In ΔABC, side AB is produced to D so that BD = BC. If ∠B = 60° and ∠A = 70°, prove that: (i) AD > CD (ii) AD > AC
Is it possible to draw a triangle with sides of length 2 cm, 3 cm and 7 cm?
In Δ ABC, ∠B = 35°, ∠C = 65° and the bisector of ∠BAC meets BC in P. Arrange AP, BP and CP in descending order.
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
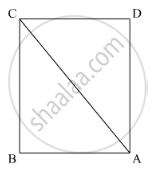
In the given figure, prove that:
CD + DA + AB + BC > 2AC
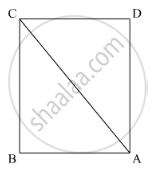
In the given figure, prove that:
CD + DA + AB > BC
Which of the following statements are true (T) and which are false (F)?
Sum of the three sides of a triangle is less than the sum of its three altitudes.
Which of the following statements are true (T) and which are false (F)?
Sum of any two sides of a triangle is greater than twice the median drawn to the third side.
Which of the following statements are true (T) and which are false (F)?
Sum of any two sides of a triangle is greater than the third side.
Which of the following statements are true (T) and which are false (F)?
Difference of any two sides of a triangle is equal to the third side.
Which of the following statements are true (T) and which are false (F)?
If two angles of a triangle are unequal, then the greater angle has the larger side opposite to it.
Which of the following statements are true (T) and which are false (F)?
Of all the line segments that can be drawn from a point to a line not containing it, the perpendicular line segment is the shortest one.
Fill in the blank to make the following statement true.
In a right triangle the hypotenuse is the .... side.
Fill in the blank to make the following statement true.
The sum of three altitudes of a triangle is ..... than its perimeter.
Fill in the blank to make the following statement true.
The sum of any two sides of a triangle is .... than the third side.
Fill in the blank to make the following statement true.
If two angles of a triangle are unequal, then the smaller angle has the........ side opposite to it.
Fill in the blank to make the following statement true.
Difference of any two sides of a triangle is........ than the third side.
Fill in the blank to make the following statement true.
If two sides of a triangle are unequal, then the larger side has .... angle opposite to it.
O is any point in the interior of ΔABC. Prove that
(i) AB + AC > OB + OC
(ii) AB + BC + CA > OA + QB + OC
(iii) OA + OB + OC >` 1/2`(AB + BC + CA)
Prove that in a quadrilateral the sum of all the sides is greater than the sum of its diagonals.
RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.7 [Page 84]
In two congruent triangles ABC and DEF, if AB = DE and BC = EF. Name the pairs of equal angles.
In two triangles ABC and DEF, it is given that ∠A = ∠D, ∠B = ∠E and ∠C =∠F. Are the two triangles necessarily congruent?
If ABC and DEF are two triangles such that AC = 2.5 cm, BC = 5 cm, ∠C = 75°, DE = 2.5 cm, DF = 5cm and ∠D = 75°. Are two triangles congruent?
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
In triangles ABC and CDE, if AC = CE, BC = CD, ∠A = 60°, ∠C = 30° and ∠D = 90°. Are two triangles congruent?
ABC is an isosceles triangle in which AB = AC. BE and CF are its two medians. Show that BE = CF.
Find the measure of each angle of an equilateral triangle.
CDE is an equilateral triangle formed on a side CD of a square ABCD. Show that ΔADE ≅ΔBCE.
Prove that the sum of three altitudes of a triangle is less than the sum of its sides.
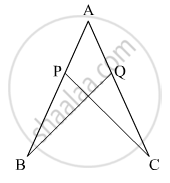
In the given figure, if AB = AC and ∠B = ∠C. Prove that BQ = CP.
RD Sharma solutions for Mathematics [English] Class 9 12 Congruent Triangles Exercise 12.8 [Pages 85 - 88]
Mark the correct alternative in each of the following:
If ABC ≅ ΔLKM, then side of ΔLKM equal to side AC of ΔABC is
LK
KM
LM
None of these
If ΔABC ≅ ΔABC is isosceles with
AB = AC
AB = BC
AC = BC
None of these
ΔPQR and ΔABC is not congruent to ΔRPQ, then which of the following is not true:
BC = PQ
AC = PR
AB = PQ
QR = BC
In triangles ABC and PQR three equality relations between some parts are as follows:
AB = QP, ∠B = ∠P and BC = PR
State which of the congruence conditions applies:
SAS
ASA
SSS
RHS
In triangles ABC and PQR, if ∠A = ∠R, ∠B = ∠P and AB = RP, then which one of the following congruence conditions applies:
SAS
ASA
SSS
RHS
In ΔPQR ≅ ΔEFD then ED =
PQ
RQ
PR
None of these
If ΔPQR≅ ΔEFD, then ∠E =
∠P
∠Q
∠R
None of these
In a ΔABC, if AB = AC and BC is produced to D such that ∠ACD = 100°, then ∠A =
20°
40°
60°
80°
In an isosceles triangle, if the vertex angle is twice the sum of the base angles, then the measure of vertex angle of the triangle is
100°
120°
110°
130°
Which of the following is not a criterion for congruence of triangles?
SAS
SSA
ASA
SSS
In the given figure, the measure of ∠B'A'C' is

50°
60°
70°
80°
If ABC and DEF are two triangles such that ΔABC \[\cong\] ΔFDE and AB = 5cm, ∠B = 40°
DF = 5cm, ∠F = 60°
DE = 5cm, ∠E = 60°
DF = 5cm, ∠E = 60°
DE = 5cm, ∠D = 40°
In the given figure, AB ⊥ BE and FE ⊥ BE. If BC = DE and AB = EF, then ΔABD is congruent to
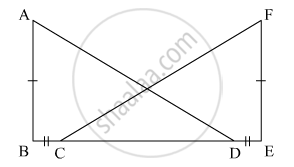
ΔEFC
ΔECF
ΔCEF
ΔFEC
In the given figure, if AE || DC and AB = AC, the value of ∠ABD is
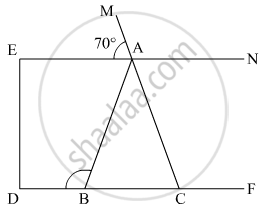
70°
110°
120°
130°
In the given figure, ABC is an isosceles triangle whose side AC is produced to E. Through C, CD is drawn parallel to BA. The value of x is

52°
76°
156°
104°
In the given figure, if AC is bisector of ∠BAD such that AB = 3 cm and AC = 5 cm, then CD =

2 cm
3 cm
4 cm
5 cm
D, E, F are the mid-point of the sides BC, CA and AB respectively of ΔABC. Then ΔDEF is congruent to triangle
ABC
AEF
BFD, CDE
AFE, BFD, CDE
ABC is an isosceles triangle such that AB = AC and AD is the median to base BC. Then, ∠BAD =
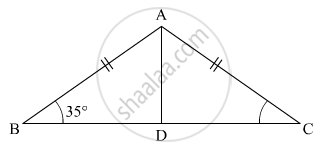
55°
70°
35°
110°
In the given figure, X is a point in the interior of square ABCD. AXYZ is also a square. If DY = 3 cm and AZ = 2 cm, then BY =

5 cm
6 cm
7 cm
8 cm
In the given figure, ABC is a triangle in which ∠B = 2∠C. D is a point on side BC such that ADbisects ∠BAC and AB = CD. BE is the bisector of ∠B. The measure of ∠BAC is
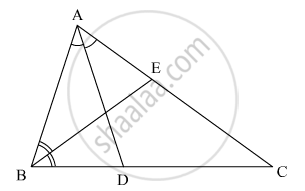
72°
73°
74°
95°
Solutions for 12: Congruent Triangles
![RD Sharma solutions for Mathematics [English] Class 9 chapter 12 - Congruent Triangles RD Sharma solutions for Mathematics [English] Class 9 chapter 12 - Congruent Triangles - Shaalaa.com](/images/8193647912-mathematics-english-class-9_6:1a030933ece146238cec338f12706a07.jpg)
RD Sharma solutions for Mathematics [English] Class 9 chapter 12 - Congruent Triangles
Shaalaa.com has the CBSE Mathematics Mathematics [English] Class 9 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. RD Sharma solutions for Mathematics Mathematics [English] Class 9 CBSE 12 (Congruent Triangles) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. RD Sharma textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 9 chapter 12 Congruent Triangles are Concept of Triangles, Properties of a Triangle, Some More Criteria for Congruence of Triangles, Inequalities in a Triangle, Criteria for Congruence of Triangles, Congruence of Triangles.
Using RD Sharma Mathematics [English] Class 9 solutions Congruent Triangles exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in RD Sharma Solutions are essential questions that can be asked in the final exam. Maximum CBSE Mathematics [English] Class 9 students prefer RD Sharma Textbook Solutions to score more in exams.
Get the free view of Chapter 12, Congruent Triangles Mathematics [English] Class 9 additional questions for Mathematics Mathematics [English] Class 9 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
