Question

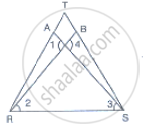
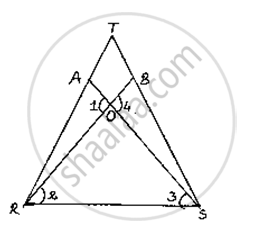
In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT 

  

Answer 1

In the figure given that 

RT = TS         ……..(1)

∠1 = 2∠2        ……..(2) 

And∠4 = `2sqrt` ................(3)

And given to prove ΔRBT≅ ΔSAT

Let the point of intersection of RB and SA be denoted by O Since RB and SA intersect at O.

∴∠AOR = ∠BOS          [Vertically opposite angles]

⇒∠1 = ∠4

⇒ 2∠2 = 2∠3                 [From (2) and (3) 

Þ

Þ ∠2 = ∠3                  ……..(4) 

Now we have RT = TS  ∠∠TRS

∴ΔTRS is an isosceles triangle  

∴∠TRS = ∠TSR            ……..(5)          [Angles opposite to equal sides are equal]   

But we have

 ∠TRS = ∠TRB + ∠2        ………(6)

And ∠TSR = ∠TSA + ∠3     ……….(7)

Putting (6) and (7) in (5) we get 

∠TRB +∠2 = ∠TSA + ∠B      

⇒∠ TRB =∠TSA       [∵  From  (4)] 

Now considerΔRBT and ΔSAT  

RT = ST              [From (1)] 

∠TRB = ∠TSA         [From (4)] 

∠RTB =∠ STA [Common angle] 

From ASA criterion of congruence, we have ΔRBT ≅ΔSAT