Advertisements
Advertisements
Question
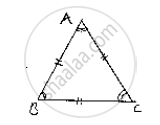
Fill the blank in the following so that the following statement is true.
In an equilateral triangle all angles are .....
Solution
In an equilateral triangle all angles are equal Reason: Since all sides are equal in a equilateral triangle, the angles opposite to equal sides will be equal
APPEARS IN
RELATED QUESTIONS
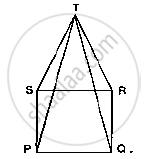
In Fig. 10.23, PQRS is a square and SRT is an equilateral triangle. Prove that
(i) PT = QT (ii) ∠TQR = 15°
In a ΔABC, if AB = AC and ∠B = 70°, find ∠A.
ABC is a triangle in which ∠B = 2 ∠C. D is a point on BC such that AD bisects ∠BAC and AB = CD.
Prove that ∠BAC = 72°.
Is it possible to draw a triangle with sides of length 2 cm, 3 cm and 7 cm?
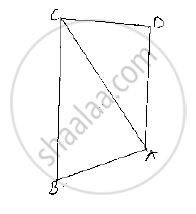
In Fig. 10.131, prove that: (i) CD + DA + AB + BC > 2AC (ii) CD + DA + AB > BC
If the angles A, B and C of ΔABC satisfy the relation B − A = C − B, then find the measure of ∠B.
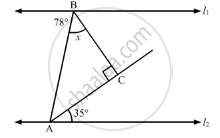
In the given figure, for which value of x is l1 || l2?
In the given figure, what is y in terms of x?

Which of the following correctly describes the given triangle?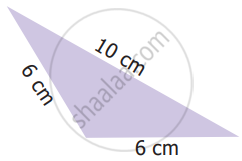
ABC is an isosceles triangle with AB = AC and D is a point on BC such that AD ⊥ BC (Figure). To prove that ∠BAD = ∠CAD, a student proceeded as follows:

In ∆ABD and ∆ACD,
AB = AC (Given)
∠B = ∠C (Because AB = AC)
and ∠ADB = ∠ADC
Therefore, ∆ABD ≅ ∆ACD (AAS)
So, ∠BAD = ∠CAD (CPCT)
What is the defect in the above arguments?
[Hint: Recall how ∠B = ∠C is proved when AB = AC].
